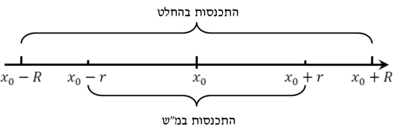את משפט 10 לא סיימנו בשיעור הקודם ולכן השלמנו זאת ב־17.5.11. חלק זה מופיע בסיכום השיעור הקודם ולא בדף הנוכחי.

התכנסות במ"ש של טורים (המשך)

דוגמה

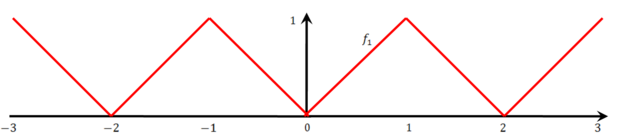
נבנה פונקציה S רציפה ב-[math]\displaystyle{ \mathbb R }[/math] שאינה גזירה באף נקודה. תחילה נגדיר [math]\displaystyle{ f_1(x)=|x| }[/math] בקטע [math]\displaystyle{ [-1,1] }[/math] עם המשך מחזורי בכל [math]\displaystyle{ \mathbb R }[/math]:

לכן [math]\displaystyle{ f_1(x+2)=f_1(x) }[/math] וכן אם [math]\displaystyle{ x\not\in\mathbb Z }[/math] אז [math]\displaystyle{ f_1'(x)\in\{\pm1\} }[/math], ואחרת הנגזרת לא קיימת. כמו כן נגדיר [math]\displaystyle{ f_2(x)=\frac14f_1(4x) }[/math] ואז [math]\displaystyle{ f_2\left(x+\frac24\right)=f_2(x) }[/math] וכן אם [math]\displaystyle{ \frac x4\not\in\mathbb Z }[/math] אז [math]\displaystyle{ f_2'(x)\in\{\pm1\} }[/math]. נמשיך להגדיר [math]\displaystyle{ f_{n+1}(x)=\frac14f_n(4x)=\frac1{4^n}f_1\left(4^nx\right) }[/math] ולכן [math]\displaystyle{ f_{n+1}\left(x+\frac 2{4^n}\right)=f_{n+1}(x) }[/math] ואם [math]\displaystyle{ \frac x{4^n}\not\in\mathbb Z }[/math] אז [math]\displaystyle{ f_{n+1}'(x)\in\{\pm1\} }[/math]. לבסוף, נגדיר [math]\displaystyle{ S(x)=\sum_{n=1}^\infty f_n(x) }[/math] אזי S רציפה ב-[math]\displaystyle{ \mathbb R }[/math] (כי כל [math]\displaystyle{ f_n }[/math] רציפה והטור מתכנס במ"ש עפ"י מבחן ה-M של וירשטרס: [math]\displaystyle{ |f_n(x)|=\left|\frac1{4^n}f_1\left(4^nx\right)\right|\le\frac1{4^n} }[/math] ו-[math]\displaystyle{ \sum\frac1{4^n} }[/math] מתכנס).

הוכחה שגויה לכך שהפונקציה לא גזירה: [math]\displaystyle{ S'(x)=\sum_{n=1}^\infty f_n'(x)=\sum_{n=1}^\infty \pm1 }[/math] שמתבדר (כי [math]\displaystyle{ \pm1\not\to0 }[/math]), ולכן הפונקציה אינה גזירה בשום נקודה. הוכחה זו אינה נכונה כי היא מתבססת על הטענה שאומרת שאם [math]\displaystyle{ f_n\to f }[/math] במ"ש ואם [math]\displaystyle{ \lim_{n\to\infty}f_n' }[/math] לא קיים אז f לא גזירה, טענה שאפשר לסתור בעזרת [math]\displaystyle{ f_n }[/math] שהגדרנו קודם: [math]\displaystyle{ \lim_{n\to\infty}f_n(x)=\lim_{n\to\infty}\frac1{4^n}f_1\left(4^nx\right)=0 }[/math] ולכן הפונקציה הגבולית (שהיא 0) גזירה, אבל [math]\displaystyle{ \lim_{n\to\infty}f_n'(x)=\lim_{n\to\infty}\frac{4^n}{4^n}f_1'(4^nx) }[/math] שמתבדר בין [math]\displaystyle{ -1 }[/math] ל-[math]\displaystyle{ 1 }[/math], עם ערכים לא מוגדרים באמצע.

הוכחה נכונה: נאמר ששתי נקודות שונות [math]\displaystyle{ x_1,x_2\in\mathbb R }[/math] מקיימות את התכונה [math]\displaystyle{ P_1 }[/math] הן נמצאות בקטע שבין שתי נקודות קיצון סמוכות של [math]\displaystyle{ f_1 }[/math] (למשל הקטע [math]\displaystyle{ [0,1] }[/math], כי הוא נמצא בין נקודות הקיצון שב-0 וב-1, או הקטע [math]\displaystyle{ [-3,-2] }[/math] וכו'). אם [math]\displaystyle{ x_1,x_2 }[/math] מקיימות זאת אזי [math]\displaystyle{ \frac{f_1(x_1)-f_1(x_2)}{x_1-x_2}\in\{\pm1\} }[/math]. נמשיך כך ונאמר ששתי נקודות [math]\displaystyle{ x_1,x_2 }[/math] מקיימות תכונה [math]\displaystyle{ P_n }[/math] אם"ם הן בקטע שבין שתי נקודות קיצון סמוכות של [math]\displaystyle{ f_n }[/math]. במקרה כזה [math]\displaystyle{ \frac{f_n(x_1)-f_n(x_2)}{x_1-x_2}\in\{\pm1\} }[/math]. נשים לב שאם הנקודות [math]\displaystyle{ x_1,x_2 }[/math] מקיימות [math]\displaystyle{ P_n }[/math] אז הן מקיימות [math]\displaystyle{ P_{n-1} }[/math], ובהכללה [math]\displaystyle{ P_n\implies P_{n-1}\implies\dots\implies P_1 }[/math]. כעת יהי [math]\displaystyle{ x\in\mathbb R }[/math] נתון ונוכיח כי [math]\displaystyle{ S'(x) }[/math] לא קיים. מספיק להוכיח שעבור סדרה [math]\displaystyle{ \{h_m\} }[/math] כלשהי כך ש-[math]\displaystyle{ 0\ne h_m\to0 }[/math] לא קיים הגבול [math]\displaystyle{ \lim_{m\to\infty}\frac{S(x+h_m)-S(x)}{h_m} }[/math]. נבחר [math]\displaystyle{ h_m=\frac2{4^m} }[/math] אם [math]\displaystyle{ x,x+\frac2{4^m} }[/math] מקיימות [math]\displaystyle{ P_m }[/math], ו-[math]\displaystyle{ h_m=-\frac2{4^m} }[/math] אחרת. נשים לב שבכל מקרה הנקודות [math]\displaystyle{ x,x+h_m }[/math] מקיימות [math]\displaystyle{ P_m }[/math] כי אם [math]\displaystyle{ x,x+\frac2{4^m} }[/math] לא מקיימות [math]\displaystyle{ P_m }[/math] אזי יש בין שתיהן נקודת קיצון של [math]\displaystyle{ f_m }[/math]. ההפרש בין שיעורי ה-x של שתי נקודות קיצון סמוכות ב-[math]\displaystyle{ f_m }[/math] הוא [math]\displaystyle{ \frac4{4^m} }[/math] ולכן [math]\displaystyle{ x,x-\frac2{4^m} }[/math] כן מקיימות [math]\displaystyle{ P_m }[/math]. כמו כן ברור כי [math]\displaystyle{ 0\ne h_m\to0 }[/math]. מתקיים [math]\displaystyle{ \forall m:\ \frac{S(x+h_m)-S(x)}{h_m}=\sum_{n=1}^\infty \frac{f_n(x+h_m)-f_n(x)}{h_m} }[/math]. כיוון ש-[math]\displaystyle{ x,x+h_m }[/math] מקיימות [math]\displaystyle{ P_m\and P_{m-1}\and\dots\and P_1 }[/math] מתקיימת לכל [math]\displaystyle{ 1\le n\le m }[/math] הטענה [math]\displaystyle{ \frac{f_n(x+h_m)-f_n(x)}{h_m}\in\{\pm1\} }[/math]. עבור [math]\displaystyle{ n\gt m }[/math] המחזור של [math]\displaystyle{ f_n }[/math] הוא [math]\displaystyle{ \frac2{4^{n-1}} }[/math]. אם [math]\displaystyle{ n\gt m }[/math] אז [math]\displaystyle{ h_m=\pm\frac2{4^m}=\pm\frac2{4^{n-1}}\cdot4^{n-m-1} }[/math] הוא מספר שלם של מחזורים, ולכן [math]\displaystyle{ f_n(x+h_m)=f_n(x) }[/math], ומכאן ש-[math]\displaystyle{ \forall n\gt m:\ \frac{f_n(x+h_m)-f_n(x)}{h_m}=0 }[/math]. לפיכך לכל m נקבל [math]\displaystyle{ \frac{S(x+h_m)-S(x)}{h_m}=\sum_{n=1}^m\pm1+\sum0 }[/math]. כאשר [math]\displaystyle{ m\to\infty }[/math] הגבול לא קיים ולכן S לא גזירה ב-x, והטענה נכונה לכל x. [math]\displaystyle{ \blacksquare }[/math]

טורי חזקות

הגדרה: טור חזקות הוא טור פונקציות מהצורה [math]\displaystyle{ \sum_{n=0}^\infty a_n(x-x_0)^n }[/math] עבור [math]\displaystyle{ a_n,x_0\in\mathbb R }[/math] לכל n. כאשר [math]\displaystyle{ \forall n:\ a_n=1 }[/math] נקבל טור הנדסי.

דוגמה: [math]\displaystyle{ \sum_{n=0}^\infty (x+5)^n }[/math] הוא טור חזקות הנדסי. אם נציב [math]\displaystyle{ y=x+5 }[/math] נקבל [math]\displaystyle{ \sum_{n=0}^\infty y^n }[/math], ולכן הטור מתכנס אם"ם [math]\displaystyle{ |y|=|x+5|\lt 1 }[/math], וסכומו הוא [math]\displaystyle{ \frac1{1-y}=-\frac1{x+4} }[/math].

משפט 1

יהי [math]\displaystyle{ \sum_{n=0}^\infty a_n(x-x_0)^n }[/math] טור חזקות כלשהו ונגדיר [math]\displaystyle{ R=\frac1{\displaystyle\limsup_{n\to\infty}\sqrt[n]{|a_n|}} }[/math] (R נקרא רדיוס ההתכנסות של הטור). אזי:

אם x מקיים [math]\displaystyle{ |x-x_0|\lt R }[/math] אז הטור מתכנס בהחלט.
אם x מקיים [math]\displaystyle{ |x-x_0|\gt R }[/math] אז הטור מתבדר.
אם [math]\displaystyle{ 0\lt r\lt R }[/math] אז הטור מתכנס במ"ש בקטע [math]\displaystyle{ [x_0-r,x_0+r] }[/math].

הוכחה

יהי x כך ש-[math]\displaystyle{ |x-x_0|\lt R }[/math] ונבחר P כך ש-[math]\displaystyle{ |x-x_0|\lt P\lt R }[/math]. מכאן נובע ש-[math]\displaystyle{ \limsup_{n\to\infty}\sqrt[n]{|a_n|}=\frac1R\lt \frac1P }[/math] ולפיכך קיים [math]\displaystyle{ n_0\in\mathbb N }[/math] כך ש-[math]\displaystyle{ \forall n\gt n_0:\ \sqrt[n]{|a_n|}\lt \frac1P }[/math]. מכאן נובע כי [math]\displaystyle{ \sqrt[n]{|a_n|}\cdot|x-x_0|\lt \frac{|x-x_0|}P\lt 1 }[/math] ולכן [math]\displaystyle{ |a_n|\cdot|x-x_0|^n\lt \left(\frac{|x-x_0|}P\right)^n\lt 1 }[/math]. מכאן שהטור [math]\displaystyle{ \sum_{n=0}^\infty \left(\frac{|x-x_0|}P\right)^n }[/math] הוא טור הנדסי שמתכנס, וממבחן ההשוואה [math]\displaystyle{ \sum_{n=0}^\infty |a_n|\cdot|x-x_0|^n }[/math] מתכנס, כלומר הטור המקורי מתכנס בהחלט בנקודה x. [math]\displaystyle{ \blacksquare }[/math]
נתון [math]\displaystyle{ |x-x_0|\gt R }[/math] ונרשום [math]\displaystyle{ P=|x-x_0| }[/math]. לפי הנתון [math]\displaystyle{ \limsup_{n\to\infty}\sqrt[n]{|a_n|}=\frac1R\gt \frac1P }[/math] ולכן יש אינסוף אינדקסים n כך ש-[math]\displaystyle{ \sqrt[n]{|a_n|}\gt \frac1P }[/math]. עבור אותם n-ים מתקיים [math]\displaystyle{ \sqrt[n]{|a_n|}\cdot|x-x_0|\gt \frac{|x-x_0|}P=1 }[/math] ולכן [math]\displaystyle{ |a_n|\cdot|x-x_0|^n\gt 1 }[/math]. לפיכך [math]\displaystyle{ \sum_{n=0}^\infty a_n(x-x_0)^n }[/math] מתבדר (כי האיבר הכללי [math]\displaystyle{ a_n(x-x_0)^n }[/math] לא שואף ל-0). [math]\displaystyle{ \blacksquare }[/math]
נבחר P כך ש-[math]\displaystyle{ 0\lt r\lt P\lt R }[/math]. כמו בסעיף 1, קיים [math]\displaystyle{ n_0\in\mathbb N }[/math] כך שלכל [math]\displaystyle{ n\gt n_0 }[/math] מתקיים [math]\displaystyle{ \sqrt[n]{|a_n|}\lt \frac1P }[/math] ולכן אם [math]\displaystyle{ |x-x_0|\le r }[/math] אז [math]\displaystyle{ \forall n\gt n_0:\ |a_n|\cdot|x-x_0|^n\lt \left(\frac rP\right)^n }[/math]. קיבלנו חסם על האיבר ה-n בטור [math]\displaystyle{ \sum_{n=0}^\infty |a_n(x-x_0)^n| }[/math] וכיוון שסכום החסמים [math]\displaystyle{ \sum_{n=0}^\infty \left(\frac rP\right)^n }[/math] הוא טור הנדסי מתכנס (כי [math]\displaystyle{ \left|\frac rP\right|\lt 1 }[/math]) נקבל ממבחן ה-M של וירשטרס ש-[math]\displaystyle{ \sum_{n=0}^\infty |a_n(x-x_0)^n| }[/math] מתכנס במ"ש ב-[math]\displaystyle{ [x_0-r,x_0+r]=\{x:\ |x-x_0|\le r\} }[/math]. [math]\displaystyle{ \blacksquare }[/math]

הערה: באופן כללי, עבור [math]\displaystyle{ L=\limsup_{n\to\infty}\sqrt[n]{|a_n|} }[/math], [math]\displaystyle{ 0\le L\le\infty }[/math]. כאשר [math]\displaystyle{ L=0 }[/math] מתקיים [math]\displaystyle{ R=\infty }[/math], ואז הטור מתכנס בהחלט לכל [math]\displaystyle{ x\in\mathbb R }[/math], ובמ"ש על כל תת קטע סופי של [math]\displaystyle{ \mathbb R }[/math]. כאשר [math]\displaystyle{ L=\infty }[/math] מתקיים [math]\displaystyle{ R=0 }[/math] ואז הטור מתכנס אך ורק כאשר [math]\displaystyle{ x=x_0 }[/math].

הערה: לא ניתן לבדוק ישירות מהמשפט התכנסות או התבדרות עבור x המקיים [math]\displaystyle{ |x-x_0|=R }[/math]. מקרה זה יש לבדוק בנפרד.