התכנסות במ"ש (המשך)[עריכה]

משפט: [math]\displaystyle{ f_n\to f }[/math] במ"ש בקטע I אם"ם לכל [math]\displaystyle{ \varepsilon\gt 0 }[/math] יש [math]\displaystyle{ n_0\in\mathbb N }[/math] כך שלכל [math]\displaystyle{ n\gt n_0 }[/math] מתקיים [math]\displaystyle{ \sup_{x\in I}|f_n(x)-f(x)|\lt \varepsilon }[/math].

דוגמה 1[עריכה]

תהי [math]\displaystyle{ f_n(x)=\ln\left(x+\frac{x^2}n\right) }[/math]. קבעו התכנסות בכל אחד מהקטעים הבאים:

[math]\displaystyle{ [a,b] }[/math] עבור [math]\displaystyle{ 0\lt a\lt b\lt \infty }[/math]
בקטע [math]\displaystyle{ (0,\infty) }[/math]

פתרון[עריכה]

פונקציית הגבול היא [math]\displaystyle{ \lim_{n\to\infty}\ln\left(x+\frac{x^2}n\right)=\ln(x+0)=\ln(x) }[/math].

נראה התכנסות במ"ש ב-[math]\displaystyle{ [a,b] }[/math]: [math]\displaystyle{ \sup_{x\in[a,b]}\left|\ln\left(x+\frac{x^2}n\right)-\ln(x)\right|=\sup_{n\in[a,b]}\left|\ln\left(1+\frac xn\right)\right|=\ln\left(1+\frac bn\right)\to0 }[/math]. [math]\displaystyle{ \blacksquare }[/math]
נראה שההתכנסות נקודתית בלבד ב-[math]\displaystyle{ (0,\infty) }[/math]: [math]\displaystyle{ \sup_{x\in(0,\infty)}|f_n(x)-f(x)|=\sup_{x\in(0,\infty)}\left|\ln\left(1+\frac xn\right)\right|=\infty }[/math]. [math]\displaystyle{ \blacksquare }[/math]

דוגמה 2[עריכה]

קבע האם [math]\displaystyle{ f_n(x)=\frac1{1+n^2x^2} }[/math] מתכנסת במ"ש ב-[math]\displaystyle{ (-\infty,\infty)=\mathbb R }[/math].

פתרון[עריכה]

קל לראות ש-[math]\displaystyle{ f(x)=\lim_{n\to\infty}f_n(x)=\begin{cases}1&x=0\\0&\text{else}\end{cases} }[/math]. נבדוק התכנסות במ"ש: [math]\displaystyle{ \sup_{x\in\mathbb R}|f_n(x)-f(x)|\ge\sup_{x\in\mathbb R}|f_n(x)|=\left|\sup_{x\in\mathbb R}\frac1{1+n^2x^2}\right|=\left|\sup_{x\in[0,\infty)}\frac1{1+n^2x^2}\right| }[/math]. נחפש מקסימום: [math]\displaystyle{ f_n'(x)=\frac{-2n^2x}{\left(1+n^2x^2\right)^2}=0 }[/math] וקל לראות שהנגזרת מתאפסת עבור [math]\displaystyle{ x=0 }[/math]. ברור ש-[math]\displaystyle{ f_n }[/math] מונוטונית יורדת ב-[math]\displaystyle{ [0,\infty) }[/math] ולכן זו אכן נקודת מקסימום גלובלית. מתקיים [math]\displaystyle{ f_n(0)=1 }[/math] ולכן [math]\displaystyle{ \sup_{x\in\mathbb R}|f_n(x)-f(x)|\ge1\not\to0 }[/math]. מכאן שההתכנסות נקודתית בלבד. [math]\displaystyle{ \blacksquare }[/math]

דוגמה 3[עריכה]

תהי [math]\displaystyle{ \{f_n\} }[/math] סדרת פונקציות המתכנסת במ"ש לפונקציה f, האם f חסומה?

פתרון[עריכה]

נבחר לדוגמה [math]\displaystyle{ f_n(x)=\frac1x+\frac1n }[/math] בקטע [math]\displaystyle{ (0,\infty) }[/math]. ברור כי [math]\displaystyle{ f(x)=\lim_{n\to\infty}f_n(x)=\frac1x }[/math] וכי אם [math]\displaystyle{ x\to0^+ }[/math] אז [math]\displaystyle{ \frac1x\to\infty }[/math], לכן f לא חסומה. [math]\displaystyle{ \blacksquare }[/math]

דוגמה 4[עריכה]

תהי [math]\displaystyle{ \{f_n\} }[/math] סדרת פונקציות המתכנסת לפונקציה f במ"ש ב-I. נוכיח כי אם כל אחת מהפונקציות [math]\displaystyle{ f_n }[/math] חסומה ב-I, אזי גם f חסומה ב-I.

פתרון[עריכה]

נרשום [math]\displaystyle{ |f|\le|f-f_n|+|f_n| }[/math]. נתון כי ההתכנסות במ"ש ולכן [math]\displaystyle{ \forall\varepsilon\gt 0:\ \exists n_0:\ \forall n\gt n_0:\ |f(x)-f_n(x)|\lt \varepsilon }[/math], בפרט עבור [math]\displaystyle{ \varepsilon=1 }[/math]. כמו כן [math]\displaystyle{ f_{n_0+1} }[/math] חסומה ב-I (מהנתון) כלומר קיים M כך ש-[math]\displaystyle{ \sup_{x\in I}|f_{n_0+1}(x)|\le M\lt \infty }[/math] ולכן מתקבל ש-[math]\displaystyle{ |f(x)|\le1+|f_{n_0+1}(x)|\lt 1+M }[/math] לכל [math]\displaystyle{ x\in I }[/math]. [math]\displaystyle{ \blacksquare }[/math]

משפט: אם [math]\displaystyle{ f_n }[/math] מתכנסת במ"ש בקטע I וכל [math]\displaystyle{ f_n }[/math] רציפה אזי f רציפה.

דוגמה 5[עריכה]

ניתן דוגמה לסדרת פונקציות רציפות המתכנסות לפונקציה רציפה אבל לא מתכנסת במ"ש.

פתרון[עריכה]

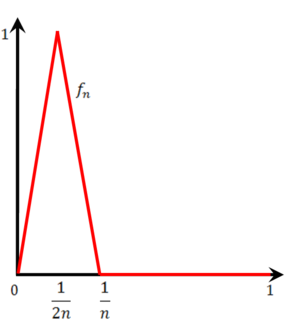
נגדיר את הפונקציה הבאה: [math]\displaystyle{ f_n(x)=\begin{cases}2nx&0\le x\le\frac1{2n}\\2-2nx&\frac1{2n}\lt x\le\frac1n\\0&\frac1n\lt x\le1\end{cases} }[/math]. קל לראות שהפונקציה הנ"ל מוגדרת בקטע [math]\displaystyle{ [0,1] }[/math], אפשר לראות שהפונקציה הנ"ל רציפה.

לכל [math]\displaystyle{ x_0 }[/math] יש [math]\displaystyle{ n_0 }[/math] כך שלכל [math]\displaystyle{ n\gt n_0 }[/math] מתקיים [math]\displaystyle{ x_0\gt \frac1{n_0} }[/math] שם מתקיים [math]\displaystyle{ f_n(x_0)=0 }[/math], כלומר [math]\displaystyle{ \{f_n(x_0)\} }[/math] סדרה קבועה מ-[math]\displaystyle{ n_0 }[/math] מסויים. כמו כן [math]\displaystyle{ \sup_{x\in[0,1]}|f_n(x)-f(x)|=1 }[/math] ולכן ההתכנסות אינה במ"ש.

טורים של פונקציות[עריכה]

דוגמה 6[עריכה]

נסמן לכל n, [math]\displaystyle{ f_n(x)=x^{n-1} }[/math] בקטע [math]\displaystyle{ (-1,1) }[/math]. מה היא פונקצית הסכום [math]\displaystyle{ S_N }[/math]?

פתרון[עריכה]

[math]\displaystyle{ S_N(x)=\sum_{n=1}^N f_n(x)=1+x+\dots+x^{N-1}=\frac{1-x^N}{1-x}\to\frac1{1-x} }[/math].

דוגמה 7[עריכה]

נוכיח כי הטור [math]\displaystyle{ S_N(x)=\sum_{n=0}^N\frac{(-1)^nx^{2n+1}}{(2n+1)!} }[/math] מתכנס ל-[math]\displaystyle{ \sin(x) }[/math] במ"ש בקטע [math]\displaystyle{ [0,2\pi] }[/math] כאשר [math]\displaystyle{ n\to\infty }[/math].

פתרון[עריכה]

בזכות טורי טיילור ברור שיש התכנסות נקודתית, נותר לבדוק התכנסות במ"ש. נסמן [math]\displaystyle{ f_n(x)=\frac{(-1)^nx^{2n+1}}{(2n+1)!} }[/math]. כאשר [math]\displaystyle{ R_N(x) }[/math] השארית בין הטור-טיילור מסדר N לבין [math]\displaystyle{ \sin }[/math] מתקיים [math]\displaystyle{ |S_N(x)-\sin(x)|=|R_N(x)|\le\left|\frac{x^{2(N+1)+1}}{(2(N+1)+1)!}\right| }[/math]. כמו כן [math]\displaystyle{ \frac{x^{2N+3}}{(2N+3)!}\le\frac{(2\pi)^{2N+3}}{(2N+3)!}=:a_N }[/math]. נוכיח ש-[math]\displaystyle{ a_N\to0 }[/math] וכך נוכיח שההתכנסות במ"ש. מספיק להסתכל על [math]\displaystyle{ \left|\frac{a_{N+1}}{a_N}\right|=\frac{(2\pi)^{2N+5}}{(2N+5)!}\frac{(2N+3)!}{(2\pi)^{2N+3}}=\frac{(2\pi)^2}{(2N+4)(2N+5)}\to0 }[/math], לכן [math]\displaystyle{ \sum_{n=1}^\infty a_n }[/math] מתכנס ובפרט [math]\displaystyle{ a_n\to0 }[/math]. [math]\displaystyle{ \blacksquare }[/math]

דוגמה 8[עריכה]

בדקו התכנסות במ"ש של [math]\displaystyle{ \sum_{n=1}^\infty \frac{\cos(nx)}{n^2} }[/math].

פתרון[עריכה]

נשים לב כי לא ידועה לנו פונקצית הגבול של הטור ולכן לא ניתן להוכיח התכנסות במ"ש ישירות מההגדרה. במקום, נפנה לתנאי קושי: יהי [math]\displaystyle{ \varepsilon\gt 0 }[/math] נתון. ברור כי [math]\displaystyle{ \left|\sum_{k=n}^m\frac{\cos(kx)}{k^2}\right|\le\left|\sum_{k=n}^m\frac1{k^2}\right| }[/math]. הטור [math]\displaystyle{ \sum_{k=1}^\infty\frac1{k^2} }[/math] מתכנס ולכן מקיים את תנאי קושי, כלומר קיים [math]\displaystyle{ n_0\in\mathbb N }[/math] כל שלכל [math]\displaystyle{ m\gt n\gt n_0 }[/math] מתקיים [math]\displaystyle{ \left|\sum_{k=n}^m\frac1{k^2}\right|\lt \varepsilon }[/math]. לכן, עבור אותו [math]\displaystyle{ n_0 }[/math], לכל [math]\displaystyle{ m\gt n\gt n_0 }[/math] מתקיים [math]\displaystyle{ \left|\sum_{k=n}^m\frac{\cos(kx)}{k^2}\right|\lt \varepsilon }[/math]. [math]\displaystyle{ \blacksquare }[/math]