משתמש:אור שחף/133 - הרצאה/1.3.11
את ההוכחה למשפט 11 לא סיימנו בשיעור הקודם ולכן השלמנו זאת ב־1.3.11. חלק זה מופיע בסיכום השיעור הקודם ולא בדף הנוכחי.
האינטגרל לפי רימן (המשך)
משפט 12 (המשפט היסודי של חשבון אינטגרלי)
תהי f מוגדרת ואינטגרבילית בקטע [math]\displaystyle{ [a,b] }[/math].
- לכל [math]\displaystyle{ x\in[a,b] }[/math] נגדיר [math]\displaystyle{ A(x)=\int\limits_a^x f }[/math]. אזי A מוגדרת היטב ורציפה ב-[math]\displaystyle{ [a,b] }[/math] ולכל [math]\displaystyle{ x_0\in[a,b] }[/math] שבה f רציפה A גזירה כך ש-[math]\displaystyle{ A'(x_0)=f(x_0) }[/math].
- נוסחת ניוטון-לייבניץ: נניח ש-f רציפה בכל הקטע [math]\displaystyle{ [a,b] }[/math]. אם F קדומה ל-f אז [math]\displaystyle{ \int\limits_a^b f=[F(x)]_{x=a}^b=F(b)-F(a) }[/math].
הוכחה
- כיוון ש-f אינטגרבילית ב-[math]\displaystyle{ [a,b] }[/math] משפט 9 נותן שלכל [math]\displaystyle{ x\in[a,b] }[/math] f אינטגרבילית בקטע [math]\displaystyle{ [a,x_0] }[/math] ולכן [math]\displaystyle{ A(x)=\int\limits_a^x f }[/math] מוגדרת היטב. נוכיח ש-A רציפה ע"י זה שהיא מקיימת את תנאי ליפשיץ. ובכן f אינטגרבילית ובפרט היא חסומה: [math]\displaystyle{ |f(x)|\le M }[/math] לכל [math]\displaystyle{ x\in[a,b] }[/math]. כעת אם [math]\displaystyle{ x,y\in[a,b] }[/math] אז [math]\displaystyle{ |A(y)-A(x)|=\left|\int\limits_a^y f-\int\limits_a^x f\right|=\left|\int\limits_x^y f\right|\le M|y-x| }[/math] ונובע ש-A רציפה. כעת נניח ש-f רציפה בנקודה [math]\displaystyle{ x_0\in[a,b] }[/math]. ר"ל A גזירה שם. ובכן [math]\displaystyle{ A(x_0+\Delta x)-A(x_0)=\int\limits_a^{x_0+\Delta x} f-\int\limits_a^{x_0} f=\int\limits_{x_0}^{x_0+\Delta x} f }[/math] ולכן [math]\displaystyle{ \frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}=\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}f }[/math]. נעיר ש-[math]\displaystyle{ \int\limits_{x_0}^{x_0+\Delta x} f(x_0)=f(x_0)\Delta x }[/math] (כי [math]\displaystyle{ f(x_0) }[/math] פונקציה קבועה). לכן [math]\displaystyle{ f(x_0)=\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}f(x_0) }[/math]. מכאן ש-[math]\displaystyle{ \frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}-f(x_0)=\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}\Big(f(t)-f(x_0)\Big)\mathrm dt }[/math]. נותר להוכיח שכאשר [math]\displaystyle{ \Delta x\to0 }[/math] אגף ימין (ולכן אגף שמאל) שואף ל-0. לצורך זה יהי [math]\displaystyle{ \varepsilon\gt 0 }[/math] נתון. כיוון ש-f רציפה ב-[math]\displaystyle{ x_0 }[/math] קיים [math]\displaystyle{ \delta\gt 0 }[/math] כך שאם [math]\displaystyle{ |t-x_0|\lt \delta }[/math] אז [math]\displaystyle{ |f(t)-f(x_0)|\lt \varepsilon }[/math]. כעת נניח ש-[math]\displaystyle{ |\Delta x|\lt \delta }[/math]. אם כן האינטגרל באגף ימין הוא על קטע בין [math]\displaystyle{ x_0 }[/math] ל-[math]\displaystyle{ x_0+\Delta x }[/math] ולכן כל t בקטע זה מקיים [math]\displaystyle{ |t-x_0|\lt \Delta x }[/math]. נובע שלכל t בקטע [math]\displaystyle{ |f(t)-f(x_0)|\lt \varepsilon }[/math]. יוצא שאם [math]\displaystyle{ |\Delta x|\le\delta }[/math] אז [math]\displaystyle{ \left|\frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}-f(x_0)\right|=\left|\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}(f(t)-f(x_0))\mathrm dt\right|\lt \frac{|\Delta x|\varepsilon}{|\Delta x|} }[/math]. הדבר נכון לכל [math]\displaystyle{ \varepsilon\gt 0 }[/math], לכן [math]\displaystyle{ \lim_{\Delta x\to0}\frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}-f(x_0)=0 }[/math] ז"א [math]\displaystyle{ A'(x_0) }[/math] קיים ושווה ל-[math]\displaystyle{ f(x_0) }[/math]. [math]\displaystyle{ \blacksquare }[/math]
- נתון ש-f רציפה בכל [math]\displaystyle{ [a,b] }[/math]. לפי החלק הקודם [math]\displaystyle{ \forall x\in[a,b]:\ A'(x)=f(x) }[/math], כלומר A קדומה ל-f ב-[math]\displaystyle{ [a,b] }[/math]. קיים קבוע c כך ש-[math]\displaystyle{ F(x)=A(x)+c }[/math] לכל [math]\displaystyle{ x\in[a,b] }[/math]. מכאן ש-[math]\displaystyle{ F(b)-F(a)=A(b)+c-\Big(A(a)+c\Big)=A(b)-A(a)=\int\limits_a^b f-\int\limits_a^a f=\int\limits_a^b f }[/math]. [math]\displaystyle{ \blacksquare }[/math]
מסקנה
אם f רציפה בקטע [math]\displaystyle{ [a,b] }[/math] אז קיימת לה פונקצייה קדומה ב-[math]\displaystyle{ [a,b] }[/math].
הוכחה
כיוון ש-f רציפה בקטע [math]\displaystyle{ [a,b] }[/math] כולו [math]\displaystyle{ A(x)=\int\limits_a^x f }[/math] קדומה ל-f ב-[math]\displaystyle{ [a,b] }[/math].
דוגמאות
- [math]\displaystyle{ f(x)=e^{x^2} }[/math]. זו פונקציה אלמנטרית ומוגדרת בכל [math]\displaystyle{ \mathbb R }[/math], ולכן רציפה שם. לפי המסקנה יש לה פונקציה קדומה. זו דוגמה קלאסית לפונקציה אלמנטרית שהפונקציה הקדומה שלה לא אלמנטרית.
- [math]\displaystyle{ e^{x^n} }[/math] כאשר [math]\displaystyle{ 1\lt n\in\mathbb N }[/math]
- [math]\displaystyle{ \frac{\sin(x)}x }[/math]
- [math]\displaystyle{ \sin(x^n) }[/math]
- [math]\displaystyle{ \cos(x^n) }[/math]
תרגילים לחידוד
- נגדיר [math]\displaystyle{ F(x)=\int\limits_2^x e^{t^3}\mathrm dt }[/math]. נמצא את [math]\displaystyle{ F'(x) }[/math]: לפי חלק א של משפט 12 מתקיים [math]\displaystyle{ F'(x)=e^{x^3} }[/math]. [math]\displaystyle{ \blacksquare }[/math]
- נגדיר [math]\displaystyle{ G(x)=\int\limits_{x^2}^{\sin(x)} e^{t^3}\mathrm dt }[/math]. נמצא את [math]\displaystyle{ G'(x) }[/math]: נגדיר [math]\displaystyle{ F(x)=\int\limits_0^x e^{t^3}\mathrm dt }[/math] ולכן [math]\displaystyle{ F'(x)=e^{x^3} }[/math]. לפי זה [math]\displaystyle{ G(x)=F(\sin(x))-F(x^2) }[/math] ולפיכך, ע"פ כלל השרשרת, [math]\displaystyle{ G'(x)=F'(\sin(x))\cos(x)-F'(x^2)\cdot2x=e^{\sin^3(x)}\cos(x)-2xe^{x^6} }[/math]. [math]\displaystyle{ \blacksquare }[/math]
הגדרה: עבור [math]\displaystyle{ f(x)\ge0 }[/math] רציפה ב-[math]\displaystyle{ [a,b] }[/math] נגדיר את השטח שמתחת לגרף של f ע"י [math]\displaystyle{ \int\limits_a^b f }[/math]. לפי זה, אם [math]\displaystyle{ f(x)\le0 }[/math] ב-[math]\displaystyle{ [a,b] }[/math] אז [math]\displaystyle{ \int\limits_a^b f }[/math] = מספר שלילי או 0 שהוא "מינוס השטח שמעל הגרף". אם f מחליפה סימן אז [math]\displaystyle{ \int\limits_a^b f }[/math] = השטח מעל ציר ה-x פחות השטח מתחת לציר ה-x ולכן [math]\displaystyle{ \int\limits_a^b |f| }[/math] = השטח בין הגרף לציר ה-x.
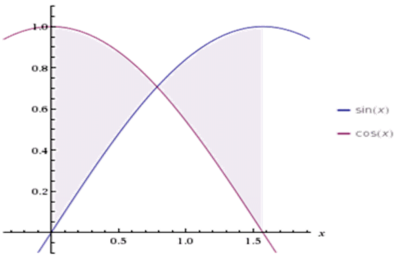
דוגמת חישוב
בגרף שמשמאל ברור שהשטח בין הגרפים הוא [math]\displaystyle{ \int\limits_a^b f-g }[/math], ובנימוק פשוט זה נכון בכל מקרה ש-[math]\displaystyle{ f(x)\ge g(x) }[/math] ב-[math]\displaystyle{ [a,b] }[/math].
למשל, נחשב את השטח שבין הגרפים [math]\displaystyle{ y=\sin(x) }[/math] ו-[math]\displaystyle{ y=\cos(x) }[/math] בקטע [math]\displaystyle{ \left[0,\tfrac\pi2\right] }[/math]:
בקטע [math]\displaystyle{ \left[0,\tfrac\pi4\right] }[/math] מתקיים [math]\displaystyle{ \cos(x)\ge\sin(x) }[/math] ובקטע [math]\displaystyle{ \left[\tfrac\pi4,\tfrac\pi2\right] }[/math] מתקיים [math]\displaystyle{ \cos(x)\le\sin(x) }[/math]. לכן השטח הוא
[math]\displaystyle{ \blacksquare }[/math]