את ההוכחה למשפט 11 לא סיימנו בהרצאה הקודמת ולכן השלמנו אותו ב-1.3.11. חלק זה מופיע בסיכום ההרצאה הקודמת ולא בדף הנוכחי.

האינטגרל לפי רימן (המשך)

משפט 12 (המשפט היסודי של חשבון אינטגרלי)

תהי f מוגדרת ואינטגרבילית בקטע [math]\displaystyle{ [a,b] }[/math].

לכל [math]\displaystyle{ x\in[a,b] }[/math] נגדיר [math]\displaystyle{ A(x)=\int\limits_a^x f }[/math]. אזי A מוגדרת היטב ורציפה ב-[math]\displaystyle{ [a,b] }[/math] ולכל [math]\displaystyle{ x_0\in[a,b] }[/math] שבה f רציפה A גזירה כך ש-[math]\displaystyle{ A'(x_0)=f(x_0) }[/math].
נוסחת ניוטון-לייבניץ: נניח ש-f רציפה בכל הקטע [math]\displaystyle{ [a,b] }[/math]. אם F קדומה ל-f אז [math]\displaystyle{ \int\limits_a^b f=[F(x)]_{x=a}^b=F(b)-F(a) }[/math].

הוכחה

כיוון ש-f אינטגרבילית ב-[math]\displaystyle{ [a,b] }[/math] משפט 9 נותן שלכל [math]\displaystyle{ x\in[a,b] }[/math] f אינטגרבילית בקטע [math]\displaystyle{ [a,x_0] }[/math] ולכן [math]\displaystyle{ A(x)=\int\limits_a^x f }[/math] מוגדרת היטב. נוכיח ש-A רציפה ע"י זה שהיא מקיימת את תנאי ליפשיץ. ובכן f אינטגרבילית ובפרט היא חסומה: [math]\displaystyle{ |f(x)|\le M }[/math] לכל [math]\displaystyle{ x\in[a,b] }[/math]. כעת אם [math]\displaystyle{ x,y\in[a,b] }[/math] אז [math]\displaystyle{ |A(y)-A(x)|=\left|\int\limits_a^y f-\int\limits_a^x f\right|=\left|\int\limits_x^y f\right|\le M|y-x| }[/math] ונובע ש-A רציפה. כעת נניח ש-f רציפה בנקודה [math]\displaystyle{ x_0\in[a,b] }[/math]. ר"ל A גזירה שם. ובכן [math]\displaystyle{ A(x_0+\Delta x)-A(x_0)=\int\limits_a^{x_0+\Delta x} f-\int\limits_a^{x_0} f=\int\limits_{x_0}^{x_0+\Delta x} f }[/math] ולכן [math]\displaystyle{ \frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}=\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}f }[/math]. נעיר ש-[math]\displaystyle{ \int\limits_{x_0}^{x_0+\Delta x} f(x_0)=f(x_0)\Delta x }[/math] (כי [math]\displaystyle{ f(x_0) }[/math] פונקציה קבועה). לכן [math]\displaystyle{ f(x_0)=\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}f(x_0) }[/math]. מכאן ש-[math]\displaystyle{ \frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}-f(x_0)=\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}\Big(f(t)-f(x_0)\Big)\mathrm dt }[/math]. נותר להוכיח שכאשר [math]\displaystyle{ \Delta x\to0 }[/math] אגף ימין (ולכן אגף שמאל) שואף ל-0. לצורך זה יהי [math]\displaystyle{ \varepsilon\gt 0 }[/math] נתון. כיוון ש-f רציפה ב-[math]\displaystyle{ x_0 }[/math] קיים [math]\displaystyle{ \delta\gt 0 }[/math] כך שאם [math]\displaystyle{ |t-x_0|\lt \delta }[/math] אז [math]\displaystyle{ |f(t)-f(x_0)|\lt \varepsilon }[/math]. כעת נניח ש-[math]\displaystyle{ |\Delta x|\lt \delta }[/math]. אם כן האינטגרל באגף ימין הוא על קטע בין [math]\displaystyle{ x_0 }[/math] ל-[math]\displaystyle{ x_0+\Delta x }[/math] ולכן כל t בקטע זה מקיים [math]\displaystyle{ |t-x_0|\lt \Delta x }[/math]. נובע שלכל t בקטע [math]\displaystyle{ |f(t)-f(x_0)|\lt \varepsilon }[/math]. יוצא שאם [math]\displaystyle{ |\Delta x|\le\delta }[/math] אז [math]\displaystyle{ \left|\frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}-f(x_0)\right|=\left|\frac1{\Delta x}\int\limits_{x_0}^{x_0+\Delta x}(f(t)-f(x_0))\mathrm dt\right|\lt \frac{|\Delta x|\varepsilon}{|\Delta x|} }[/math]. הדבר נכון לכל [math]\displaystyle{ \varepsilon\gt 0 }[/math], לכן [math]\displaystyle{ \lim_{\Delta x\to0}\frac{A(x_0+\Delta x)-A(x_0)}{\Delta x}-f(x_0)=0 }[/math] ז"א [math]\displaystyle{ A'(x_0) }[/math] קיים ושווה ל-[math]\displaystyle{ f(x_0) }[/math]. [math]\displaystyle{ \blacksquare }[/math]
נתון ש-f רציפה בכל [math]\displaystyle{ [a,b] }[/math]. לפי החלק הקודם [math]\displaystyle{ \forall x\in[a,b]:\ A'(x)=f(x) }[/math], כלומר A קדומה ל-f ב-[math]\displaystyle{ [a,b] }[/math]. קיים קבוע c כך ש-[math]\displaystyle{ F(x)=A(x)+c }[/math] לכל [math]\displaystyle{ x\in[a,b] }[/math]. מכאן ש-[math]\displaystyle{ F(b)-F(a)=A(b)+c-\Big(A(a)+c\Big)=A(b)-A(a)=\int\limits_a^b f-\int\limits_a^a f=\int\limits_a^b f }[/math]. [math]\displaystyle{ \blacksquare }[/math]

מסקנה

אם f רציפה בקטע [math]\displaystyle{ [a,b] }[/math] אז קיימת לה פונקצייה קדומה ב-[math]\displaystyle{ [a,b] }[/math].

הוכחה

כיוון ש-f רציפה בקטע [math]\displaystyle{ [a,b] }[/math] כולו [math]\displaystyle{ A(x)=\int\limits_a^x f }[/math] קדומה ל-f ב-[math]\displaystyle{ [a,b] }[/math].

דוגמאות

[math]\displaystyle{ f(x)=e^{x^2} }[/math]. זו פונקציה אלמנטרית ומוגדרת בכל [math]\displaystyle{ \mathbb R }[/math], ולכן רציפה שם. לפי המסקנה יש לה פונקציה קדומה. זו דוגמה קלאסית לפונקציה אלמנטרית שהפונקציה הקדומה שלה לא אלמנטרית.
[math]\displaystyle{ e^{x^n} }[/math] כאשר [math]\displaystyle{ 1\lt n\in\mathbb N }[/math]
[math]\displaystyle{ \frac{\sin(x)}x }[/math]
[math]\displaystyle{ \sin(x^n) }[/math]
[math]\displaystyle{ \cos(x^n) }[/math]

תרגילים לחידוד

נגדיר [math]\displaystyle{ F(x)=\int\limits_2^x e^{t^3}\mathrm dt }[/math]. נמצא את [math]\displaystyle{ F'(x) }[/math]: לפי חלק א של משפט 12 מתקיים [math]\displaystyle{ F'(x)=e^{x^3} }[/math]. [math]\displaystyle{ \blacksquare }[/math]
נגדיר [math]\displaystyle{ G(x)=\int\limits_{x^2}^{\sin(x)} e^{t^3}\mathrm dt }[/math]. נמצא את [math]\displaystyle{ G'(x) }[/math]: נגדיר [math]\displaystyle{ F(x)=\int\limits_0^x e^{t^3}\mathrm dt }[/math] ולכן [math]\displaystyle{ F'(x)=e^{x^3} }[/math]. לפי זה [math]\displaystyle{ G(x)=F(\sin(x))-F(x^2) }[/math] ולפיכך, ע"פ כלל השרשרת, [math]\displaystyle{ G'(x)=F'(\sin(x))\cos(x)-F'(x^2)\cdot2x=e^{\sin^3(x)}\cos(x)-2xe^{x^6} }[/math]. [math]\displaystyle{ \blacksquare }[/math]

הגדרה: עבור [math]\displaystyle{ f(x)\ge0 }[/math] רציפה ב-[math]\displaystyle{ [a,b] }[/math] נגדיר את השטח שמתחת לגרף של f ע"י [math]\displaystyle{ \int\limits_a^b f }[/math]. לפי זה, אם [math]\displaystyle{ f(x)\le0 }[/math] ב-[math]\displaystyle{ [a,b] }[/math] אז [math]\displaystyle{ \int\limits_a^b f }[/math] = מספר שלילי או 0 שהוא "מינוס השטח שמעל הגרף". אם f מחליפה סימן אז [math]\displaystyle{ \int\limits_a^b f }[/math] = השטח מעל ציר ה-x פחות השטח מתחת לציר ה-x ולכן [math]\displaystyle{ \int\limits_a^b |f| }[/math] = השטח בין הגרף לציר ה-x.

דוגמת חישוב

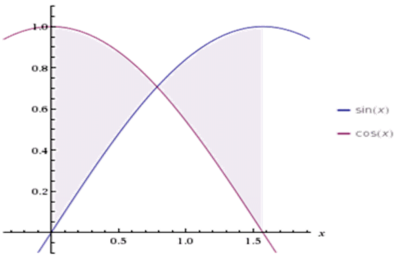
בגרף שמשמאל ברור שהשטח בין הגרפים הוא [math]\displaystyle{ \int\limits_a^b f-g }[/math], ובנימוק פשוט זה נכון בכל מקרה ש-[math]\displaystyle{ f(x)\ge g(x) }[/math] ב-[math]\displaystyle{ [a,b] }[/math].

למשל, נחשב את השטח שבין הגרפים [math]\displaystyle{ y=\sin(x) }[/math] ו-[math]\displaystyle{ y=\cos(x) }[/math] בקטע [math]\displaystyle{ \left[0,\tfrac\pi2\right] }[/math]:

בקטע [math]\displaystyle{ \left[0,\tfrac\pi4\right] }[/math] מתקיים [math]\displaystyle{ \cos(x)\ge\sin(x) }[/math] ובקטע [math]\displaystyle{ \left[\tfrac\pi4,\tfrac\pi2\right] }[/math] מתקיים [math]\displaystyle{ \cos(x)\le\sin(x) }[/math]. לכן השטח הוא

[math]\displaystyle{ \begin{align}\int\limits_0^\frac\pi2 |\cos(x)-\sin(x)|\mathrm dx&=\int\limits_0^\frac\pi4 \Big(\cos(x)-\sin(x)\Big)\mathrm dx+\int\limits_\frac\pi4^\frac\pi2 \Big(\sin(x)-\cos(x)\Big)\mathrm dx\\&=\left([\sin(x)+\cos(x)]_{x=0}^\frac\pi4\right)+\left([-\sin(x)-\cos(x)]_{x=\frac\pi4}^\frac\pi2\right)\\&=\left(\frac\sqrt22+\frac\sqrt22-(0+1)\right)+\left(-1-0+\frac\sqrt22+\frac\sqrt22\right)\\&=2\sqrt2-2\end{align} }[/math]

[math]\displaystyle{ \blacksquare }[/math]