את דוגמה 4 לא סיימנו בשיעור הקודם ולכן השלמנו זאת ב־31.5.11. חלק זה מופיע בסיכום השיעור הקודם ולא בדף הנוכחי.

טורי חזקות (המשך)

תזכורת: בהרצאה הקודמת הוכחנו ש-[math]\displaystyle{ \arctan(x)=\sum_{n=0}^\infty(-1)^n\frac{x^{2n+1}}{2n+1} }[/math] לכל [math]\displaystyle{ x\in(-1,1) }[/math] והערנו שאם ניתן להציב [math]\displaystyle{ x=1 }[/math] נקבל את המשוואה היפה [math]\displaystyle{ \frac\pi4=\sum_{n=0}^\infty\frac{(-1)^n}{2n+1} }[/math]. כמו כן אמרנו ש-[math]\displaystyle{ \ln(x)=\sum_{n=0}^\infty(-1)^n\frac{(x-1)^{n+1}}{n+1} }[/math] עבור [math]\displaystyle{ x\in(0,2) }[/math] ושאם מותר להציב [math]\displaystyle{ x=2 }[/math] אזי [math]\displaystyle{ \ln(2)=\sum_{n=0}^\infty\frac{(-1)^n}{n+1} }[/math].

משפט 5 (משפט אבל)

נניח ש-[math]\displaystyle{ f(x)=\sum_{n=0}^\infty a_nx^n }[/math] בקטע [math]\displaystyle{ (-1,1) }[/math] ו-[math]\displaystyle{ \sum_{n=0}^\infty a_n }[/math] מתכנס ל-[math]\displaystyle{ S\in\mathbb R }[/math], אזי [math]\displaystyle{ \lim_{x\to1^-}f(x) }[/math] קיים ושווה ל-S.

הוכחה

נעזר בסכימה בחלקים: נסמן [math]\displaystyle{ S_N=\sum_{n=0}^N a_n }[/math] ולכן [math]\displaystyle{ \forall N\in\mathbb N:\ \sum_{n=0}^Na_nx^n=\sum_{n=0}^{N-1}S_n\left(x^n-x^{n+1}\right)+S_Nx^N }[/math] כאשר [math]\displaystyle{ -1\lt x\lt 1 }[/math]. לפי הנתון [math]\displaystyle{ S=\lim_{N\to\infty}S_N }[/math], ולכן אם [math]\displaystyle{ 0\lt x\lt 1 }[/math] אז [math]\displaystyle{ \lim_{N\to\infty}S_Nx^N=0 }[/math] ועבור [math]\displaystyle{ 0\le x\le 1 }[/math] מתקיים [math]\displaystyle{ f(x)=\sum_{n=0}^\infty a_nx^n=\sum_{n=0}^\infty S_n\left(x^n-x^{n+1}\right)=(1-x)\sum_{n=0}^\infty S_nx^n }[/math]. כמו כן, [math]\displaystyle{ \forall x\in[0,1):\ 1=(1-x)\sum_{n=0}^\infty x^n }[/math] (כי [math]\displaystyle{ \frac1{1-x}=\sum_{n=0}^\infty x^n }[/math]). לכן [math]\displaystyle{ S=(1-x)\sum_{n=0}^\infty Sx^n }[/math] ומכאן שעבור [math]\displaystyle{ x\in[0,1) }[/math] מתקיים [math]\displaystyle{ f(x)-S=(1-x)\sum_{n=0}^\infty(S_n-S)x^n }[/math]. נרצה להוכיח ש-[math]\displaystyle{ \lim_{x\to1^-}f(x)-S=0 }[/math]: יהי [math]\displaystyle{ \varepsilon\gt 0 }[/math] נתון ומכיוון ש-[math]\displaystyle{ \lim_{n\to\infty}S_n=S }[/math] קיים [math]\displaystyle{ n_0\in\mathbb N }[/math] כך שלכל [math]\displaystyle{ n\gt n_0 }[/math] יתקיים [math]\displaystyle{ |S_n-S|\lt \frac\varepsilon2 }[/math]. נסמן [math]\displaystyle{ I_1=(1-x)\sum_{n=0}^{n_0}(S_n-S)x^n }[/math] וכן [math]\displaystyle{ I_2=(1-x)\sum_{n=n_0+1}^\infty(S_n-S)x^n }[/math], לכן [math]\displaystyle{ f(x)-S=I_1+I_2 }[/math]. עתה [math]\displaystyle{ |I_2|\le(1-x)\sum_{n=n_0+1}^\infty|S_n-S|x^n\lt \frac\varepsilon2(1-x)\sum_{n=n_0+1}^\infty x^n\le\frac\varepsilon2(1-x)\sum_{n=0}^\infty x^n=\frac\varepsilon2 }[/math]. לגבי [math]\displaystyle{ I_1 }[/math] נגדיר [math]\displaystyle{ M=\sum_{n=0}^{n_0}|S_n-S| }[/math] ולכן [math]\displaystyle{ |I_1|\le(1-x)\sum_{n=0}^{n_0}|S_n-S| }[/math]. עתה [math]\displaystyle{ x\to1^- }[/math] ולכן [math]\displaystyle{ 0\lt 1-x\lt \frac\varepsilon{2M} }[/math], לכן [math]\displaystyle{ |I_1|\le\frac\varepsilon{2M}M=\frac\varepsilon2 }[/math]. לסיכום הוכחנו שאם [math]\displaystyle{ 1-\frac\varepsilon{2M}\lt x\lt 1 }[/math] אזי [math]\displaystyle{ |f(x)-S|\lt |I_1|+|I_2|\lt \varepsilon }[/math] ולכן [math]\displaystyle{ \lim_{x\to1^-}f(x)-S=0 }[/math]. [math]\displaystyle{ \blacksquare }[/math]

מסקנה

לגבי טור חזקות כללי [math]\displaystyle{ f(x)=\sum_{n=0}^\infty a_n(x-x_0)^n }[/math] בעל רדיוס התכנסות R:

אם [math]\displaystyle{ \sum_{n=0}^\infty a_nR^n }[/math] מתכנס ל-S אזי [math]\displaystyle{ \lim_{x\to x_0+R^-}f(x) }[/math] קיים ושווה ל-S.
אם [math]\displaystyle{ \sum_{n=0}^\infty a_n(-R)^n }[/math] מתכנס ל-T אזי [math]\displaystyle{ \lim_{x\to(x_0-R)^+}f(x) }[/math] קיים ושווה ל-T.

הוכחה

נציב [math]\displaystyle{ y=\frac{x-x_0}R }[/math] ולכן [math]\displaystyle{ f(x)=\sum_{n=0}^\infty \left(a_nR^n\right)y^n }[/math] עבור [math]\displaystyle{ |x-x_0|\lt R }[/math], כלומר עבור [math]\displaystyle{ |y|\lt 1 }[/math]. נגדיר [math]\displaystyle{ g(y)=f(x) }[/math] ולכן מתקיימים תנאי משפט אבל ומתקיים [math]\displaystyle{ \lim_{y\to1^-}g(y)=S }[/math], לכן [math]\displaystyle{ \lim_{x\to x_0+R^-}f(x)=S }[/math]. [math]\displaystyle{ \blacksquare }[/math]
נציב [math]\displaystyle{ y=\frac{x-x_0}{-R} }[/math] ונוכיח כמו בסעיף 1. [math]\displaystyle{ \blacksquare }[/math]

משפט 6 (משפט דיני)

נניח שלכל n [math]\displaystyle{ f_n }[/math] רציפה ב-[math]\displaystyle{ [a,b] }[/math] ונניח שסדרת הפונקציות מונוטונית, כלומר לכל [math]\displaystyle{ x\in[a,b] }[/math] הסדרה [math]\displaystyle{ \{f_n(x)\}_{n=1}^\infty }[/math] עולה או לכל [math]\displaystyle{ x\in[a,b] }[/math] הסדרה [math]\displaystyle{ \{f_n(x)\}_{n=1}^\infty }[/math] יורדת. כמו כן ידוע כי [math]\displaystyle{ f_n\to f }[/math] ו-f רציפה ב-[math]\displaystyle{ [a,b] }[/math], אזי ההתכנסות במ"ש.

הסבר

לפני ההוכחה נסביר למה צריך את כל הנתונים:

אם הקטע פתוח במקום סגור, נבחר את הקטע [math]\displaystyle{ (0,1) }[/math] ואת סדרת הפונקציות [math]\displaystyle{ f_n(x)=x^n }[/math]. ברור כי כל הפונקציות רציפות בקטע וסדרת הפונקציות מונוטונית, וכן הפונקציה הגבולית היא הפונקציה הרציפה [math]\displaystyle{ f(x)=0 }[/math], אבל כבר הוכחנו בעבר שההתכנסות אינה במ"ש.
בקטע סגור [math]\displaystyle{ [0,1] }[/math] נבחר באותה סדרת פונקציות. הפונקציה הגבולית היא [math]\displaystyle{ f(x)=\begin{cases}0&0\le x\lt 1\\1&x=1\end{cases} }[/math] שאינה רציפה, ואכן ההתכנסות אינה במ"ש.
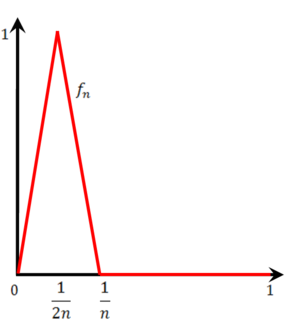
נגדיר סדרת פונקציות לפי הגרף שמשמאל. כל [math]\displaystyle{ f_n }[/math] רציפה ב-[math]\displaystyle{ [0,1] }[/math] והן מתכנסות לפונקציה הרציפה 0, אבל סדרת הפונקציות לא מונוטונית, ואכן ההתכנסות אינה במ"ש.
נגדיר [math]\displaystyle{ f_n(x)=\begin{cases}x^n&0\le x\lt 1\\0&x=1\end{cases} }[/math] ולכן סדרת הפונקציות מונוטונית אבל הפונקציות אינן רציפות, ואכן, למרות שהפונקציה הגבולית 0 רציפה, ההתכנסות אינה במ"ש.

הוכחה

במקרה הראשון נניח שסדרת הפונקציות יורדת מונוטונית. לכן [math]\displaystyle{ \{f_n-f\} }[/math] היא סדרת פונקציות יורדת מונוטונית השואפת ל-0 ב-[math]\displaystyle{ [a,b] }[/math]. נסמן [math]\displaystyle{ g_n=f_n-f }[/math] (ולכן [math]\displaystyle{ g_n }[/math] חיובית) ונניח בשלילה שההתכנסות [math]\displaystyle{ g_n\to0 }[/math] אינה במ"ש בקטע. לפיכך קיים [math]\displaystyle{ \varepsilon\gt 0 }[/math] כל שלכל [math]\displaystyle{ n_0\in\mathbb N }[/math] קיימים [math]\displaystyle{ n\gt n_0 }[/math] ו-[math]\displaystyle{ x\in[a,b] }[/math] עבורם [math]\displaystyle{ g_n(x)\gt \varepsilon }[/math]. בפרט, עבור [math]\displaystyle{ n_0=1 }[/math] קיימים [math]\displaystyle{ n_1\gt n_0 }[/math] ו-[math]\displaystyle{ x_1\in[a,b] }[/math] כך ש-[math]\displaystyle{ g_{n_1}(x_1)\gt \varepsilon }[/math]. עבור [math]\displaystyle{ n_0=n_1+1 }[/math] קיימים [math]\displaystyle{ n_2\gt n_0 }[/math] ו-[math]\displaystyle{ x_2\in[a,b] }[/math] כך ש-[math]\displaystyle{ g_{n_2}(x_2)\gt \varepsilon }[/math] וכן הלאה. בדרך זו בונים תת סדרה [math]\displaystyle{ \{g_{n_k}\} }[/math] של [math]\displaystyle{ \{g_n\} }[/math] וסדרה [math]\displaystyle{ \{x_k\} }[/math] ב-[math]\displaystyle{ [a,b] }[/math] כך ש-[math]\displaystyle{ \forall k:\ g_{n_k}(x_k)\gt \varepsilon }[/math]. [math]\displaystyle{ \{x_k\} }[/math] נמצאת ב-[math]\displaystyle{ [a,b] }[/math] ולכן היא חסומה, אזי לפי משפט בולצאנו ויירשראס יש תת סדרה [math]\displaystyle{ \{x_{k_l}\} }[/math] מתכנסת, נאמר ל-[math]\displaystyle{ x_0\in[a,b] }[/math]. לפי הבנייה הנ"ל מתקיים [math]\displaystyle{ \forall l:\ g_{n_{k_l}}(x_{k_l})\gt \varepsilon }[/math] ומכיוון ש-[math]\displaystyle{ \lim_{l\to\infty} g_{n_{k_l}}(x_0)=0 }[/math] קיים [math]\displaystyle{ l_0\in\mathbb N }[/math] כך שלכל [math]\displaystyle{ l\gt l_0 }[/math] יתקיים [math]\displaystyle{ g_{n_{k_l}}(x_0)\lt \frac\varepsilon2 }[/math]. [math]\displaystyle{ g_{n_{k_{l_0+1}}} }[/math] פונקציה רציפה שקטנה מ-[math]\displaystyle{ \frac\varepsilon2 }[/math] ב-[math]\displaystyle{ x_0 }[/math] ולכן יש סביבה S של [math]\displaystyle{ x_0 }[/math] שבה [math]\displaystyle{ g_{n_{k_{l_0+1}}} }[/math] קטנה מ-[math]\displaystyle{ \varepsilon }[/math]. ה-[math]\displaystyle{ g_n }[/math] יורדות ולכן לכל [math]\displaystyle{ l\gt l_0 }[/math] ולכל [math]\displaystyle{ x\in S }[/math] מתקיים [math]\displaystyle{ g_{n_{k_l}}(x)\lt \varepsilon }[/math], אבל לפי הבנייה [math]\displaystyle{ x_{k_l}\to x_0 }[/math] ולכן לכל l מספיק גדול מתקיים [math]\displaystyle{ g_{n_{k_l}}(x_{k_l})\lt \varepsilon }[/math], בסתירה לכך שלכל l מתקיים [math]\displaystyle{ g_{n_{k_l}}(x_{k_l})\gt \varepsilon }[/math]. הסתירה מוכיחה את המשפט במקרה הזה.

במקרה השני נניח שסדרת הפונקציות עולה מונוטונית. נסמן [math]\displaystyle{ g_n=-f_n }[/math] ולכן [math]\displaystyle{ \{g_n\} }[/math] יורדת ומתקיימים שאר תנאי המשפט, ולכן [math]\displaystyle{ g_n\to -f }[/math] במ"ש. מכאן ש-[math]\displaystyle{ f_n\to f }[/math] במ"ש והוכחנו גם את המקרה השני. [math]\displaystyle{ \blacksquare }[/math]

השתנות חסומה

אינטואיטיבית, פונקציה בעלת השתנות חסומה היא פונקציה שהשינוי שלה בציר ה-y הוא סופי (הגדרה מדוייקת ניתן בהרצאה הבאה).

דוגמאות

[math]\displaystyle{ \sin(x) }[/math]
ההשתנות הכללית של [math]\displaystyle{ \sin }[/math] בקטע [math]\displaystyle{ \left[0,\tfrac32\pi\right] }[/math] היא 3 (ובפרט היא חסומה) כי הפונקציה עלתה 1 וירדה 2.
על [math]\displaystyle{ [1,\infty) }[/math] ההשתנות של [math]\displaystyle{ \frac1x }[/math] היא 1 כי הפונקציה ירדה מ-1 ל-0.
לפונקציה [math]\displaystyle{ \sin\left(\frac1x\right) }[/math] ב-[math]\displaystyle{ (0,1) }[/math] יש השתנות אינסופית כי היא עלתה וירדה בין [math]\displaystyle{ \pm1 }[/math] אינסוף פעמים.
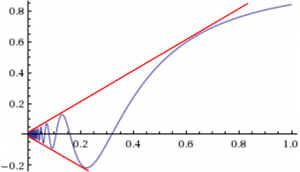
[math]\displaystyle{ x\sin\left(\frac1x\right) }[/math]
באדום: [math]\displaystyle{ y=\pm x }[/math]
נגדיר [math]\displaystyle{ f(x)=x\sin\left(\frac1x\right) }[/math] בקטע [math]\displaystyle{ (0,1) }[/math]. האם יש לה השתנות חסומה? כאן יותר קשה לנחש מה ההשתנות כי מחד יש אינסוף עליות ומורדות, ומצד שני כאשר [math]\displaystyle{ x\to0^+ }[/math] גם גדלי העליות והירידות שואפים ל-0. נוכיח שההשתנות אינה חסומה: לכל [math]\displaystyle{ n\in\mathbb N }[/math] יש לפונקציה נקודת קיצון ב-[math]\displaystyle{ \frac1{\pi n+\pi/2} }[/math], וב-[math]\displaystyle{ \left[\tfrac1{\pi(n+1)+\pi/2},\tfrac1{\pi n+\pi/2}\right] }[/math] היא עולה או יורדת מהקו [math]\displaystyle{ y=\pm x }[/math] ל-[math]\displaystyle{ y=\mp x }[/math], לכן ההשתנות שלה בקטע זה היא [math]\displaystyle{ \frac1{\pi(n+1)+\pi/2}+\frac1{\pi n+\pi/2} }[/math], שגדול מ-[math]\displaystyle{ \frac2{\pi(n+1)+\pi/2} }[/math]. מכאן נובע שההשתנות הכוללת ב-[math]\displaystyle{ (0,1) }[/math] גדולה מ-[math]\displaystyle{ \sum_{n=1}^\infty \frac2{\pi(n+1)+\pi/2}=\infty }[/math], ומכאן של-f אין השתנות חסומה ב-[math]\displaystyle{ (0,1) }[/math]. [math]\displaystyle{ \blacksquare }[/math]