מבחנים ופתרונות

סרטוני ותקציר ההרצאות

פלייליסט של כל הסרטונים

פלייליסט ההרצאות של אינפי 1 למדמח תשפ"א

פרק 1 - מספרים וחסמים

קבוצות מספרים

הטבעיים [math]\displaystyle{ \mathbb{N}=\{1,2,3,...\} }[/math]
השלמים [math]\displaystyle{ \mathbb{Z}=\{0,-1,1,-2,2,...\} }[/math]
הרציונאליים [math]\displaystyle{ \mathbb{Q}=\left\{\frac{p}{n}|p\in\mathbb{Z},n\in\mathbb{N}\right\} }[/math]
הממשיים [math]\displaystyle{ \mathbb{R} }[/math], כל השברים העשרוניים כולל האינסופיים

העשרה: בנייה של שדה הממשיים באמצעות חתכי דדקינד

לא קיים [math]\displaystyle{ x\in\mathbb{Q} }[/math] כך ש [math]\displaystyle{ x^2=2 }[/math].
במילים פשוטות, [math]\displaystyle{ \sqrt{2} }[/math] אינו רציונאלי (בהמשך נוכיח שיש מספר ממשי כזה).

חזקות ולוגריתמים

לכל מספר ממשי [math]\displaystyle{ x\in\mathbb{R} }[/math] ולכל מספר טבעי [math]\displaystyle{ n\in\mathbb{N} }[/math] נגדיר [math]\displaystyle{ x^n=x\cdots x }[/math] כפל n פעמים
לכל מספר ממשי אי שלילי [math]\displaystyle{ 0\leq x\in\mathbb{R} }[/math] ולכל מספר טבעי [math]\displaystyle{ n\in\mathbb{N} }[/math] נגדיר [math]\displaystyle{ x^{\frac{1}{n}}=\sqrt[n]{x} }[/math] כלומר המספר האי שלילי שבחזקת n שווה לx.
לכל מספר ממשי אי שלילי [math]\displaystyle{ 0\leq x\in\mathbb{R} }[/math] ולכל זוג מספרים טבעיים [math]\displaystyle{ n,k\in\mathbb{N} }[/math] נגדיר [math]\displaystyle{ x^{\frac{n}{k}}=\sqrt[k]{x^n} }[/math]
לכל מספר ממשי [math]\displaystyle{ x\in\mathbb{R} }[/math] נגדיר [math]\displaystyle{ x^0=1 }[/math]

מה לגבי חזקות ממשיות אי רציונליות?
נגדיר אותן באמצעות גבול של חזקות רציונאליות

לכל מספר ממשי [math]\displaystyle{ x\in\mathbb{R} }[/math] ולכל חזקה ממשית שלילית [math]\displaystyle{ -a\lt 0 }[/math] נגדיר [math]\displaystyle{ x^{-a}=\frac{1}{x^a} }[/math]

לכל [math]\displaystyle{ 0\lt a\neq 1 }[/math] נגדיר את [math]\displaystyle{ log_a(x) }[/math] להיות המספר שa בחזקתו שווה לx.
חוקי לוגים:
- [math]\displaystyle{ log_a(x)+log_a(y)=log_a(xy) }[/math]
- [math]\displaystyle{ log_a(x^y)=y log_a(x) }[/math]
- [math]\displaystyle{ \log_a(x)=\frac{log_b(x)}{log_b(a)} }[/math]

חסמים

תהי [math]\displaystyle{ A\subseteq \mathbb{R} }[/math] אזי:
- [math]\displaystyle{ M\in\mathbb{A} }[/math] נקרא המקסימום של A או האיבר הגדול ביותר של A אם לכל [math]\displaystyle{ a\in A }[/math] מתקיים כי [math]\displaystyle{ a\leq M }[/math]
- [math]\displaystyle{ M\in\mathbb{R} }[/math] נקרא חסם מלעיל של A אם לכל [math]\displaystyle{ a\in A }[/math] מתקיים כי [math]\displaystyle{ a\leq M }[/math]
- [math]\displaystyle{ m\in\mathbb{A} }[/math] נקרא המינימום של A או האיבר הקטן ביותר של A אם לכל [math]\displaystyle{ a\in A }[/math] מתקיים כי [math]\displaystyle{ a\geq m }[/math]
- [math]\displaystyle{ m\in\mathbb{R} }[/math] נקרא חסם מלרע של A אם לכל [math]\displaystyle{ a\in A }[/math] מתקיים כי [math]\displaystyle{ a\geq m }[/math]

כמו כן:
- אם יש איבר קטן ביותר בקבוצת חסמי המלעיל של A הוא נקרא החסם העליון של A, או הסופרמום של A ומסומן [math]\displaystyle{ \sup(A) }[/math]
- אם יש איבר גדול ביותר בקבוצת חסמי המלרע של A הוא נקרא החסם התחתון של A, או האינפימום של A ומסומן [math]\displaystyle{ \inf(A) }[/math]

בשדה הממשיים לכל קבוצה לא ריקה וחסומה מלעיל יש חסם עליון, ולכל קבוצה לא ריקה וחסומה מלרע יש חסם תחתון.
בשדה הרציונאליים זה לא נכון; לקבוצה [math]\displaystyle{ A=\{x\in\mathbb{Q}|x^2\lt 2\} }[/math] אין מספר רציונאלי קטן ביותר מבין חסמי המלעיל שלה.

תהי [math]\displaystyle{ A\subseteq \mathbb{R} }[/math] ויהי [math]\displaystyle{ M\in\mathbb{R} }[/math] אזי:
- M הוא החסם העליון של A אם ורק אם M הוא חסם מלעיל של A ולכל מספר [math]\displaystyle{ M-\varepsilon\lt M }[/math] קיים מספר [math]\displaystyle{ a\in A }[/math] כך ש [math]\displaystyle{ a\gt M-\varepsilon }[/math]
- m הוא החסם התחתון של A אם ורק אם m הוא חסם מלרע של A ולכל מספר [math]\displaystyle{ m\lt m+\varepsilon }[/math] קיים מספר [math]\displaystyle{ a\in A }[/math] כך ש [math]\displaystyle{ a\lt m+\varepsilon }[/math]

דוגמא: תהיינה [math]\displaystyle{ \emptyset\neq A,B\subseteq\mathbb{R} }[/math] חסומות מלעיל כך שA אינה מכילה חסמי מלעיל של B, אזי [math]\displaystyle{ \sup(A)\leq\sup(B) }[/math]

שיטות הוכחה בסיסיות

שיטות הוכחה בסיסיות

הוכחת טענות מכומתות - טענות 'לכל' וטענות 'קיים'

פרק 2 - סדרות

הגדרת הגבול

הגדרת הגבול של סדרה:
תהי סדרה ממשית [math]\displaystyle{ a_n }[/math] ויהי מספר ממשי [math]\displaystyle{ L\in\mathbb{R} }[/math].
[math]\displaystyle{ L }[/math] הינו גבול הסדרה [math]\displaystyle{ a_n }[/math] (מסומן [math]\displaystyle{ \lim a_n=L }[/math] או [math]\displaystyle{ a_n\to L }[/math]) אם:
- לכל סביבה של הגבול, קיים מקום בסדרה שאחריו כל איברי הסדרה נמצאים בסביבה הנתונה, כלומר:
- לכל מרחק [math]\displaystyle{ \varepsilon\gt 0 }[/math] קיים מקום [math]\displaystyle{ N\in\mathbb{N} }[/math] כך שאחריו לכל [math]\displaystyle{ n\gt N }[/math] מתקיים כי [math]\displaystyle{ |a_n-L|\lt \varepsilon }[/math]

נגדיר ש[math]\displaystyle{ a_n\to\infty }[/math] אם לכל [math]\displaystyle{ M\gt 0 }[/math] קיים [math]\displaystyle{ N\in\mathbb{N} }[/math] כך שלכל [math]\displaystyle{ n\gt N }[/math] מתקיים כי [math]\displaystyle{ a_n\gt M }[/math]
נגדיר ש[math]\displaystyle{ a_n\to -\infty }[/math] אם [math]\displaystyle{ -a_n\to\infty }[/math]

טענה: תהי [math]\displaystyle{ a_n\to \infty }[/math] אזי [math]\displaystyle{ \frac{1}{a_n}\to 0 }[/math]
טענה: תהי [math]\displaystyle{ 0\neq a_n\to 0 }[/math] אזי [math]\displaystyle{ \frac{1}{|a_n|}\to\infty }[/math]

אם [math]\displaystyle{ a_n\to L_1 }[/math] וכן [math]\displaystyle{ a_n\to L_2 }[/math] אזי [math]\displaystyle{ L_1=L_2 }[/math]

סדרה המתכנסת לגבול סופי חסומה.

[math]\displaystyle{ a_n\to L \iff a_{n+1}\to L }[/math]
בפרט, כל שינוי, תוספת או החסרה של מספר סופי של איברים לא משפיע על גבול הסדרה.

תהי סדרה[math]\displaystyle{ a_n }[/math] המתכנסת לגבול סופי והמקיימת לכל [math]\displaystyle{ n }[/math] כי [math]\displaystyle{ a\lt a_n }[/math] אזי [math]\displaystyle{ \lim a_n\geq a }[/math]

שאיפה לאפס

תהי סדרה [math]\displaystyle{ a_n }[/math] ויהי [math]\displaystyle{ L\in\mathbb{R} }[/math] אזי [math]\displaystyle{ a_n\to L }[/math] אם ורק אם [math]\displaystyle{ |a_n-L|\to 0 }[/math]
- בפרט [math]\displaystyle{ a_n\to 0 }[/math] אם ורק אם [math]\displaystyle{ |a_n|\to 0 }[/math]

תהי [math]\displaystyle{ a_n\to 0 }[/math] ותהי [math]\displaystyle{ b_n }[/math] חסומה, אזי [math]\displaystyle{ a_nb_n\to 0 }[/math]

תהיינה [math]\displaystyle{ a_n,b_n\to 0 }[/math] אזי גם [math]\displaystyle{ a_n+b_n\to 0 }[/math]

משפטי סנדביץ'

משפט הסנדביץ' -
- תהיינה סדרות המקיימות לכל n כי [math]\displaystyle{ a_n\leq b_n \leq c_n }[/math]
- כמו כן, יהי [math]\displaystyle{ L\in\mathbb{R} }[/math] כך ש [math]\displaystyle{ a_n,c_n\to L }[/math]
- אזי [math]\displaystyle{ b_n\to L }[/math]
חצי סנדביץ'-
- תהיינה סדרות המקיימות לכל n כי [math]\displaystyle{ a_n\leq b_n }[/math]
- כמו כן נתון כי [math]\displaystyle{ a_n\to\infty }[/math]
- אזי [math]\displaystyle{ b_n\to \infty }[/math]
חצי סנדביץ' על הרצפה -
- תהיינה סדרות המקיימות לכל n כי [math]\displaystyle{ |a_n|\leq b_n }[/math]
- כמו כן נתון כי [math]\displaystyle{ b_n\to 0 }[/math]
- אזי [math]\displaystyle{ a_n\to 0 }[/math]

מבוא לחשבון גבולות (אריתמטיקה של גבולות)

תהיינה [math]\displaystyle{ b_n\to L_b\in \mathbb{R} }[/math], [math]\displaystyle{ a_n\to L_a\in \mathbb{R} }[/math] אזי
- [math]\displaystyle{ a_n+b_n\to L_a+L_b }[/math]
- [math]\displaystyle{ a_n\cdot b_n \to L_a\cdot L_b }[/math]
- אם [math]\displaystyle{ L_b\neq 0 }[/math] אזי [math]\displaystyle{ \frac{a_n}{b_n}\to\frac{L_a}{L_b} }[/math]

אינדוקציה

משפט האינדוקציה המתמטית
תהי סדרת טענות כך שמתקיימים שני התנאים הבאים:
- הטענה הראשונה נכונה.
- לכל [math]\displaystyle{ n\in \mathbb{N} }[/math] אם הטענה הn מתקיימת אז גם הטענה הn+1 מתקיימת.
אזי כל הטענות בסדרה נכונות

אי שיוויון ברנולי: יהי [math]\displaystyle{ -1\lt x\in\mathbb{R} }[/math] אזי לכל [math]\displaystyle{ n\in\mathbb{N} }[/math] מתקיים כי [math]\displaystyle{ (1+x)^n\geq 1+nx }[/math]

חזקת אינסוף

תהי [math]\displaystyle{ 0\lt a_n\to a }[/math] אזי:
- אם [math]\displaystyle{ a\gt 1 }[/math] מתקיים כי [math]\displaystyle{ (a_n)^n \to \infty }[/math]
- אם [math]\displaystyle{ a\lt 1 }[/math] מתקיים כי [math]\displaystyle{ (a_n)^n\to 0 }[/math]
שימו לב כי ייתכן ו[math]\displaystyle{ 1\lt a_n\to 1 }[/math], כלומר איברי הסדרה גדולים מ1 אך גבולה הוא 1 ואז המשפט אינו תקף.

כלל המנה

כלל המנה (הוכחה בסיכום הבא על אי-שוויון הממוצעים).
- תהי סדרה [math]\displaystyle{ a_n }[/math] המקיימת כי גבול המנה הוא [math]\displaystyle{ \left|\frac{a_{n+1}}{a_n}\right|\to L }[/math] אזי:
  - אם [math]\displaystyle{ 1\lt L\leq\infty }[/math] מתקיים כי [math]\displaystyle{ |a_n|\to\infty }[/math]
  - אם [math]\displaystyle{ 0\leq L\lt 1 }[/math] מתקיים כי [math]\displaystyle{ a_n\to 0 }[/math]
  - מתקיים כי [math]\displaystyle{ \sqrt[n]{|a_n|}\to L }[/math]

דוגמאות:
- [math]\displaystyle{ \frac{n}{2^n}\to 0 }[/math]
- [math]\displaystyle{ \sqrt[n]{n}\to 1 }[/math]
- עבור [math]\displaystyle{ a\gt 0 }[/math] מתקיים [math]\displaystyle{ \sqrt[n]{a}\to 1 }[/math]
- [math]\displaystyle{ \sqrt[n]{n!}\to \infty }[/math]

חזקות של גבולות

יהי [math]\displaystyle{ 0\lt a\in\mathbb{R} }[/math] ותהי [math]\displaystyle{ b_n\to 0 }[/math] אזי [math]\displaystyle{ a^{b_n}\to 1 }[/math]
- רעיון הוכחה: אם [math]\displaystyle{ a\geq 1 }[/math] אזי [math]\displaystyle{ a^{-\frac{1}{m}}\leq a^{b_n}\leq a^{\frac{1}{m}} }[/math] והרי [math]\displaystyle{ \sqrt[m]{a}\to 1 }[/math] לפי כלל המנה

יהי [math]\displaystyle{ 0\lt a\in\mathbb{R} }[/math] ותהי [math]\displaystyle{ b_n\to L\in \mathbb{R} }[/math] אזי [math]\displaystyle{ a^{b_n}\to a^L }[/math]
- רעיון הוכחה: [math]\displaystyle{ a^{b_n} = a^{b_n-L}\cdot a^L\to 1\cdot a^L }[/math]

תהי [math]\displaystyle{ a_n\to 1 }[/math] ותהי [math]\displaystyle{ b_n\to L\in\mathbb{R} }[/math] אזי [math]\displaystyle{ a_n^{b_n}\to 1 }[/math]
- רעיון הוכחה:[math]\displaystyle{ a_n^{[L]-1}\leq a_n^{b_n}\leq a_n^{[L]+1} }[/math] לפי חשבון גבולות (כפל) שני הצדדים שואפים ל1. (אם [math]\displaystyle{ a_n\lt 1 }[/math] אי השיוויון הפוך).

תהי [math]\displaystyle{ a_n\to a\gt 0 }[/math] ותהי [math]\displaystyle{ b_n\to L\in\mathbb{R} }[/math] אזי [math]\displaystyle{ a_n^{b_n}\to a^L }[/math]
- רעיון הוכחה: [math]\displaystyle{ a_n^{b_n}=\left(\frac{a_n}{a}\right)^{b_n} \cdot a^{b_n} \to 1\cdot a^L }[/math]

תהי [math]\displaystyle{ 0\leq a_n\to 0 }[/math] ותהי [math]\displaystyle{ b_n\to L\gt 0 }[/math] אזי [math]\displaystyle{ a_n^{b_n}\to 0 }[/math]
- רעיון הוכחה: החל משלב מסויים [math]\displaystyle{ 0\leq a_n^{b_n}\leq \frac{1}{m^{\frac{L}{2}}} }[/math]

סדרות מונוטוניות והמספר e

כל סדרה מונוטונית הינה חסומה מתכנסת לגבול סופי, או שאינה חסומה ושואפת לגבול אינסופי.

דוגמא: נביט בסדרה [math]\displaystyle{ a_1\gt 0,\ a_{n+1}=a_n^2+a_n }[/math]
- כיוון ש [math]\displaystyle{ a_{n+1}-a_n=a_n^2\geq 0 }[/math] מדובר בסדרה מונוטונית עולה.
- אם הסדרה חסומה:
  - קיים לה גבול סופי [math]\displaystyle{ a_n\to L }[/math]
  - נחשב את גבול שני צידי המשוואה [math]\displaystyle{ a_{n+1}=a_n^2+a_n }[/math]
  - לכן [math]\displaystyle{ L=L^2+L }[/math] ולכן [math]\displaystyle{ L=0 }[/math]
  - אבל הסדרה עולה וחסומה מלמטה ע"י האיבר הראשון ולכן [math]\displaystyle{ L\geq a_1 }[/math]
  - כלומר [math]\displaystyle{ L=0\lt a_1\leq L }[/math] בסתירה.
- מכאן הסדרה אינה חסומה, וכיוון שהיא עולה [math]\displaystyle{ a_n\to\infty }[/math]

המספר e (הוכחות בעזרת אי-שוויון הממוצעים).

[math]\displaystyle{ 2\lt e\lt 4 }[/math].

תתי סדרות וגבולות חלקיים

הגדרת גבול חלקי

לכל סדרת מקומות [math]\displaystyle{ k_n\in\mathbb{N} }[/math] המקיימת לכל [math]\displaystyle{ n }[/math] כי [math]\displaystyle{ k_n\lt k_{n+1} }[/math] נגדיר כי [math]\displaystyle{ a_{k_n} }[/math] הינה תת סדרה של הסדרה [math]\displaystyle{ a_n }[/math]
שימו לב כי מקומות תת הסדרה הם באותו הסדר כמו בסדרה המקורית, ואסור לחזור על איבר פעמיים.

לדוגמא:
- נביט בסדרה [math]\displaystyle{ a_n=(-1)^n }[/math]
- אזי [math]\displaystyle{ a_{2n}=(-1)^{2n}=1 }[/math] היא תת הסדרה של האיברים במקומות הזוגיים [math]\displaystyle{ k_n=2n }[/math]

נגדיר ש[math]\displaystyle{ L }[/math] הוא גבול חלקי של הסדרה [math]\displaystyle{ a_n }[/math] אם קיימת תת סדרה [math]\displaystyle{ a_{k_n} }[/math] כך ש [math]\displaystyle{ a_{k_n}\to L }[/math]

טענה - יהי [math]\displaystyle{ L }[/math] סופי או אינסופי, אזי:
- [math]\displaystyle{ a_n\to L }[/math] אם ורק אם לכל תת סדרה [math]\displaystyle{ a_{k_n} }[/math] מתקיים כי [math]\displaystyle{ a_{k_n}\to L }[/math]

משפט בולצאנו-ויירשטראס

לכל סדרה יש תת סדרה מונוטונית.

משפט בולצאנו-ויירשטראס - לכל סדרה חסומה יש תת סדרה מתכנסת.

גבול עליון וגבול תחתון

תהי סדרה [math]\displaystyle{ a_n }[/math]
נגדיר את הגבול העליון שלה (limsup):
- אם [math]\displaystyle{ a_n }[/math] אינה חסומה מלעיל אזי [math]\displaystyle{ \overline{\lim}a_n=\infty }[/math]
- אם [math]\displaystyle{ a_n }[/math] חסומה מלעיל ויש לה גבול חלקי סופי כלשהו, נגדיר את [math]\displaystyle{ \overline{\lim}a_n }[/math] להיות החסם העליון של קבוצת הגבולות החלקיים של הסדרה
- אחרת, נגדיר [math]\displaystyle{ \overline{\lim}a_n=-\infty }[/math]
נגדיר את הגבול התחתון שלה (liminf):
- אם [math]\displaystyle{ a_n }[/math] אינה חסומה מלרע אזי [math]\displaystyle{ \underline{\lim}a_n=-\infty }[/math]
- אם [math]\displaystyle{ a_n }[/math] חסומה מלרע ויש לה גבול חלקי סופי כלשהו, נגדיר את [math]\displaystyle{ \underline{\lim}a_n }[/math] להיות החסם התחתון של קבוצת הגבולות החלקיים של הסדרה
- אחרת, נגדיר [math]\displaystyle{ \underline{\lim}a_n=\infty }[/math]

לכל גבול חלקי L של הסדרה מתקיים כי:
[math]\displaystyle{ \underline{\lim}a_n\leq L\leq \overline{\lim}a_n }[/math]

הגבול העליון והגבול התחתון הם גבולות חלקיים (כלומר יש תת סדרה ששואפת לגבול העליון, ויש תת סדרה ששואפת לגבול התחתון).

לכל [math]\displaystyle{ -\infty\leq L\leq \infty }[/math] מתקיים כי [math]\displaystyle{ a_n \to L }[/math] אם ורק אם [math]\displaystyle{ \underline{\lim}a_n=\overline{\lim}a_n=L }[/math]

תתי סדרות המכסות סדרה

אם ניתן לחלק סדרה למספר סופי של תתי סדרות המכסות את כולה, וכולן שואפות לאותו הגבול - אזי הסדרה כולה שואפת לגבול זה.
ייתכן שניתן לחלק סדרה לאינסוף תתי סדרות שכולם שואפות לאותו הגבול, אך הסדרה לא תשאף לגבול זה.

כלל הe

אם [math]\displaystyle{ a_n\to\infty }[/math] אזי [math]\displaystyle{ \left(1+\frac{1}{a_n}\right)^{a_n}\to e }[/math]
- [math]\displaystyle{ [a_n]\leq a_n \leq [a_n]+1 }[/math], כאשר [math]\displaystyle{ [a_n] }[/math] הוא המספר השלם הגדול ביותר שקטן או שווה ל[math]\displaystyle{ a_n }[/math].
- [math]\displaystyle{ \left(1+\frac{1}{[a_n]+1}\right)^{[a_n]}\leq\left(1+\frac{1}{a_n}\right)^{a_n}\leq \left(1+\frac{1}{[a_n]}\right)^{[a_n]+1} }[/math]
- שני הצדדים שואפים לe ולכן לפי כלל הסנדוויץ הסדרה אכן שואפת לe.
אם [math]\displaystyle{ a_n\to -\infty }[/math] אזי [math]\displaystyle{ \left(1+\frac{1}{a_n}\right)^{a_n}\to e }[/math]
- ראשית [math]\displaystyle{ \left(1-\frac{1}{n}\right)^{n}\to \frac{1}{e} }[/math] (הוכחה בקישור לערך על המספר e).
- כעת חזקה שלילית הופכת את השבר, וניתן לסיים את ההוכחה באופן דומה להוכחה במקרה הקודם.

אם [math]\displaystyle{ a_n\to 1 }[/math] אזי [math]\displaystyle{ a_n^{b_n}\to e^{\lim b_n\cdot(a_n-1)} }[/math]
- [math]\displaystyle{ a_n^{b_n}=\left[\left(1+(a_n-1)\right)^{\frac{1}{a_n-1}}\right]^{ b_n\cdot (a_n-1)} }[/math].
- [math]\displaystyle{ \left(1+(a_n-1)\right)^{\frac{1}{a_n-1}}\to e }[/math] בין אם [math]\displaystyle{ a_n-1 }[/math] שלילי או חיובי, לפי הטענות לעיל.
- שימו לב שאם [math]\displaystyle{ a_n=1 }[/math], אז ממילא מקבלים 1 בנוסחא הסופית, ואז לא צריך לחלק ב[math]\displaystyle{ a_n-1 }[/math] ששווה אפס.

דוגמא:
- [math]\displaystyle{ \lim\left(\frac{n+1}{n-2}\right)^n=e^{\lim n\cdot\left(\frac{n+1}{n-2}-1\right)}=e^{\lim\frac{3n}{n-2}}=e^3 }[/math]

חשבון גבולות (אריתמטיקה של גבולות)

אריתמטיקה מורחבת (הכתיב הוא מקוצר ואינו מדוייק):
- חסומה כפול אפיסה = אפיסה
- חסומה חלקי אינסוף = אפיסה
- [math]\displaystyle{ \infty+\infty=\infty }[/math]
- [math]\displaystyle{ \infty\cdot\infty=\infty }[/math]
- [math]\displaystyle{ \infty^\infty=\infty }[/math]
- [math]\displaystyle{ \frac{1}{0}\neq\infty }[/math]
- [math]\displaystyle{ \frac{1}{0^+}=\infty }[/math]
- [math]\displaystyle{ 0^\infty = 0 }[/math]
- אינסוף כפול סדרה השואפת למספר חיובי = אינסוף.
- אינסוף כפול סדרההשואפת למספר שלילי = אינסוף.
- יש גבול סופי + אין גבול סופי = אין גבול סופי.
- אינסוף ועוד חסומה שווה אינסוף.
- אם [math]\displaystyle{ a\gt 1 }[/math] אזי [math]\displaystyle{ a^\infty=\infty }[/math]
- חזקת סדרות שואפת לחזקת הגבולות.
- אינסוף בחזקת מספר חיובי זה אינסוף

המקרים הבעייתיים

המקרים הבעייתיים בהם צריך להפעיל מניפולציות אלגבריות או משפטים על מנת לחשב את הגבול:
- [math]\displaystyle{ \frac{0}{0},\frac{\infty}{\infty},0\cdot\infty,\infty-\infty,0^0,\infty^0,1^\infty }[/math]

פרק 3 - טורים

פרק 4 - פונקציות ורציפות

מבוא לגבולות

מבוא לגבולות (שיטות אלגבריות: כפל בצמוד, הוצאת חזקה משמעותית).
- [math]\displaystyle{ \lim_{x\to 2}\frac{x^2-4}{x-2} }[/math]
- [math]\displaystyle{ \lim_{x\to\infty}\frac{2x^2+5x+3}{3x^2-100} }[/math]
- [math]\displaystyle{ \lim_{x\to \infty}\sqrt{x^2+1}-x }[/math]
- [math]\displaystyle{ \lim_{x\to \infty}\sqrt{x^2+x+1}-x }[/math]
- [math]\displaystyle{ \lim_{x\to\infty}x^2-x }[/math]

הגדרת הגבול לפי קושי

הגדרת הגבול לפי היינה

מרבית כללי האריתמטיקה המורחבות נובעים "בחינם" עבור פונקציות

אם ניתן לחלק סדרה לתתי סדרות שכולן מתכנסות לאותו גבול, אזי זה גבול הסדרה.
מסקנה: גבול של פונקציה קיים בנקודה אם"ם הגבולות החד צדדיים קיימים ושווים לו.

הפונקציות הטריגונומטריות

הגדרת סינוס וקוסינוס ע"י מעגל היחידה.
- [math]\displaystyle{ sin^2(x)+cos^2(x)=1 }[/math]
- [math]\displaystyle{ sin(-x)=-sin(x),cos(-x)=cos(x) }[/math]
- [math]\displaystyle{ sin(a+b)=sin(a)cos(b)+sin(b)cos(a),cos(a+b)=cos(a)cos(b)-sin(a)sin(b) }[/math]
- [math]\displaystyle{ sin(2x)=2sin(x)cos(x),cos(2x)=cos^2(x)-sin^2(x) }[/math]

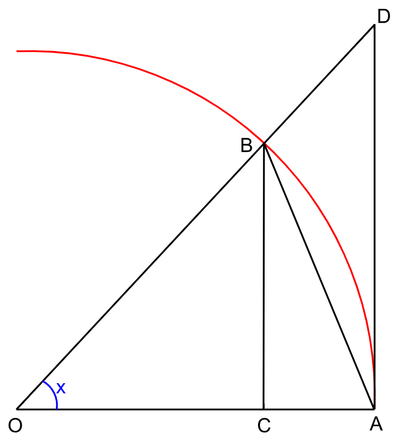
- עבור זוית [math]\displaystyle{ 0\lt x\lt \frac{\pi}{2} }[/math] שטח המשולש חסום בשטח הגזרה (משולש פיצה עם הקשה) שחסום בשטח המשולש:
- [math]\displaystyle{ S_{\triangle AOB}\lt S_{\bigcirc AOB}\lt S_{\triangle AOD} }[/math]
- [math]\displaystyle{ \frac{sin(x)}{2}\lt \frac{x}{2}\lt \frac{tan(x)}{2} }[/math]
  - כיוון ש[math]\displaystyle{ 0\lt sin(x)\lt x }[/math] בתחום [math]\displaystyle{ (0,\frac{\pi}{2}) }[/math], נובע לפי סנדוויץ' ש[math]\displaystyle{ \lim_{x\to 0^+}sin(x)=0 }[/math].
  - כיוון שמדובר בפונקציה אי זוגית, נובע שזה גם הגבול משני הצדדים.
  - כעת בתחום [math]\displaystyle{ (-\frac{\pi}{2},\frac{\pi}{2}) }[/math] הקוסינוס חיובית ולכן [math]\displaystyle{ cos(x)=\sqrt{1-sin^2(x)} }[/math] ונובע כי [math]\displaystyle{ \lim_{x\to 0}cos(x)=1 }[/math].
- נחלק את אי השיוויון הטריגונומטרי בסינוס ונקבל:
- [math]\displaystyle{ 1\lt \frac{x}{sin(x)}\lt \frac{1}{cos(x)} }[/math]
- לפי כלל הסנדביץ [math]\displaystyle{ \lim_{x\to 0^+}\frac{sin(x)}{x}=1 }[/math]
- כיוון שמדובר בפונקציה זוגית, נובע שהגבול משני הצדדים שווה 1.

ראינו ש[math]\displaystyle{ \lim_{x\to 0}\frac{sin(x)}{x}=1 }[/math].
שימו לב ש[math]\displaystyle{ \lim_{x\to\infty}\frac{sin(x)}{x}=0 }[/math], כיוון שמדובר בחסומה חלקי שואפת לאינסוף.

רציפות

גבול של הרכבת פונקציות נכשל ללא רציפות.
- [math]\displaystyle{ f(x)=\frac{x}{x}, g(x)=0 }[/math] מתקיים כי [math]\displaystyle{ \lim_{x\to 0}f(x)=1,\lim_{x\to 2}g(x)=0 }[/math] אבל [math]\displaystyle{ \lim_{x\to 2}f(g(x))\neq 1 }[/math].

רציפות.
הגדרה:
פונקציה f נקראית רציפה בקטע [math]\displaystyle{ [a,b] }[/math] אם f רציפה בכל נקודה בקטע [math]\displaystyle{ (a,b) }[/math] ובנוסף [math]\displaystyle{ \lim_{x\to a^+}f(x)=f(a) }[/math] וגם [math]\displaystyle{ \lim_{x\to b^-}f(x)=f(b) }[/math]

טענה: אם f רציפה ב[math]\displaystyle{ x_0 }[/math] אזי לכל סדרה [math]\displaystyle{ x_n\to x_0 }[/math] (גם אם אינה שונה מ[math]\displaystyle{ x_0 }[/math]) מתקיים כי [math]\displaystyle{ f(x_n)\to f(x_0) }[/math].
הרכבת רציפות: תהי f רציפה ב[math]\displaystyle{ x_0 }[/math] ותהי g רציפה ב[math]\displaystyle{ f(x_0) }[/math]. אזי [math]\displaystyle{ g\circ f }[/math] רציפה ב[math]\displaystyle{ x_0 }[/math].
- הוכחה:
- תהי סדרה [math]\displaystyle{ x_0\neq x_n\to x_0 }[/math] אזי [math]\displaystyle{ f(x_n)\to f(x_0) }[/math]
- לפי הטענה הקודמת, [math]\displaystyle{ g(f(x_n))\to g(f(x_0)) }[/math].

מיון אי רציפות.
- רציפות - הגבול בנקודה שווה לערך בנקודה.
- סליקה - הגבול קיים וסופי בנקודה, אך שונה מהערך בנקודה או שהפונקציה אינה מוגדרת בנקודה.
- קפיצתית (מין ראשון) - הגבולות החד צדדיים קיימים סופיים ושונים בנקודה.
- עיקרית (מין שני) - אחד הגבולות החד צדדיים אינו קיים או שאינו סופי.

פרק 5 - גזירות

הגדרת הנגזרת

[math]\displaystyle{ f'(x)=\lim_{h\to 0}\frac{f(x+h)-f(x)}{h} }[/math]
[math]\displaystyle{ \lim{h\to 0} \frac{f(x_0+h)-f(x_0)}{h} =\{h=x-x_0\} = \lim_{x\to x_0}\frac{f(x)-f(x_0)}{x-x_0} }[/math]
- הסבר לגבי שיטת ההצבה בה השתמשנו לעיל:
- נניח כי [math]\displaystyle{ \lim{h\to 0} \frac{f(x_0+h)-f(x_0)}{h}=f'(x_0) }[/math] ונוכיח כי [math]\displaystyle{ \lim_{x\to x_0}\frac{f(x)-f(x_0)}{x-x_0}=f'(x_0) }[/math], והוכחה דומה בכיוון ההפוך.
- תהי [math]\displaystyle{ x_0\neq x_n\to x_0 }[/math] נגדיר את הסדרה [math]\displaystyle{ 0\neq h_n=x_n-x_0\to 0 }[/math].
- כיוון ש[math]\displaystyle{ \frac{f(x_0+h_n)-f(x_0)}{h_n}\to f'(x_0) }[/math] נובע כי [math]\displaystyle{ \frac{f(x_n)-f(x_0)}{x_n-x_0}\to f'(x_0) }[/math].
אם f גזירה בנקודה, היא רציפה בנקודה:
- צ"ל [math]\displaystyle{ \lim_{x\to x_0}f(x)=f(x_0) }[/math]
- לפי אריתמטיקה של גבולות זה שקול ל [math]\displaystyle{ \lim_{x\to x_0}f(x)-f(x_0)=0 }[/math]
- לפי עקרון win (קיצור של wouldn't it be nice?) מתקיים כי [math]\displaystyle{ \lim_{x\to x_0}f(x)-f(x_0)=\lim_{x\to x_0}\frac{f(x)-f(x_0)}{x-x_0}\cdot (x-x_0)=f'(x_0)\cdot 0 = 0 }[/math]
פונקציה הערך המוחלט אינה גזירה באפס
- [math]\displaystyle{ (|x|)'(0) = \lim_{h\to 0}\frac{|h|-|0|}{h}=\lim\frac{|h|}{h} }[/math] וגבול זה אינו קיים, כיוון שהגבולות החד צדדים שונים.
- ניתן לשים לב גם ש[math]\displaystyle{ |x|=\sqrt{x^2} }[/math], וכמו כן נראה בהמשך כי[math]\displaystyle{ \sqrt{x} }[/math] אינה גזירה באפס.

הנגזרות של הפונקציות האלמנטריות

טריגו:
- [math]\displaystyle{ \lim_{h\to 0}\frac{1-cos(h)}{h}=\lim_{h\to 0}\frac{sin^2(h)}{h(1+cos(h))}=\lim_{h\to 0}sin(h)\cdot \frac{sin(h)}{h}\cdot \frac{1}{1+cos(h)}=0\cdot 1 \cdot \frac{1}{2}=0 }[/math]
- [math]\displaystyle{ (sin(x))'=\lim_{h\to 0}\frac{sin(x+h)-sin(x)}{h}=\lim_{h\to 0}\frac{sin(x)cos(h)+sin(h)cos(x)-sin(x)}{h}=\lim_{h\to 0}sin(x)\cdot \frac{cos(h)-1}{h} + cos(x)\cdot \frac{sin(h)}{h}=cos(x) }[/math]
- באופן דומה [math]\displaystyle{ (cos(x))'=-sin(x) }[/math]
לוג:
- [math]\displaystyle{ \lim_{h\to 0}\frac{log(1+h)}{h}=\lim_{h\to 0}\frac{1}{h}\cdot log(1+h)=\lim_{h\to 0}log\left(\left(1+h\right)^{\frac{1}{h}}\right)=log(e) }[/math]
  - המעבר האחרון נובע מהעובדה שפונקצית הלוג רציפה.
  - (בפרט נובע כי [math]\displaystyle{ \lim_{x\to 0}\frac{ln(1+x)}{x}=1 }[/math].)
- [math]\displaystyle{ (log(x))'=\lim_{h\to 0}\frac{log(x+h)-log(x)}{h}= \lim_{h\to 0}\frac{log\left(\frac{x+h}{x}\right)}{h}=\lim_{h\to 0}\frac{1}{x}\cdot\frac{log\left(1+\frac{h}{x}\right)}{\frac{h}{x}}=\frac{log(e)}{x} }[/math]
  - בפרט נובע כי [math]\displaystyle{ (ln(x))' = \frac{1}{x} }[/math]
אקספוננט:
- [math]\displaystyle{ \lim_{h\to 0}\frac{a^h-1}{h} = \{t=a^h-1, h=log_a(1+t)\} = \lim_{t\to 0} \frac{t}{log_a(1+t)} = \frac{1}{log_a(e)} = \frac{1}{\frac{ln(e)}{ln(a)}}=ln(a) }[/math]
- [math]\displaystyle{ (a^x)' = \lim_{h\to 0}\frac{a^{x+h}-a^x}{h}= \lim_{h\to 0}a^x\cdot \frac{a^h-1}{h}=a^x\cdot ln(a) }[/math]
  - בפרט נובע כי [math]\displaystyle{ (e^x)'=e^x }[/math].
חזקה:
- [math]\displaystyle{ (x^\alpha)'=\alpha x^{\alpha-1} }[/math] לכל [math]\displaystyle{ \alpha\in \mathbb{R} }[/math], הוכחה בהמשך.
  - בפרט:
  - [math]\displaystyle{ (1)'=0 }[/math]
  - [math]\displaystyle{ (\frac{1}{x})' = (x^{-1})'=-\frac{1}{x^2} }[/math]
  - [math]\displaystyle{ (\sqrt{x})'=(x^{\frac{1}{2}})'=\frac{1}{2\sqrt{x}} }[/math]

תהי f גזירה ב[math]\displaystyle{ x_0 }[/math] ותהי g הגזירה ב[math]\displaystyle{ f(x_0) }[/math]:

[math]\displaystyle{ (g\circ f)'(x_0) = \lim_{x\to x_0} \frac{g(f(x))-g(f(x_0))}{x-x_0} }[/math]
תהי סדרה [math]\displaystyle{ x_0\neq x_n\to x_0 }[/math].
רוצים לומר ש[math]\displaystyle{ \frac{g(f(x_n))-g(f(x_0))}{x_n-x_0}= \frac{g(f(x_n))-g(f(x_0))}{f(x_n)-f(x_0)}\cdot \frac{f(x_n)-f(x_0)}{x_n-x_0}\to g'(f(x_0))\cdot f'(x_0) }[/math].
אמנם [math]\displaystyle{ f(x_n)\to f(x_0) }[/math] בגלל שהרציפות נובעת מהגזירות, אבל לא ידוע ש[math]\displaystyle{ f(x_n)\neq f(x_0) }[/math] ובמקרה זה אנחנו כופלים ומחלקים באפס.
אם יש תת סדרה [math]\displaystyle{ a_n }[/math] של [math]\displaystyle{ x_n }[/math] עבורה [math]\displaystyle{ f(a_n)=f(x_0) }[/math] אזי [math]\displaystyle{ \frac{f(a_n)-f(x_0)}{a_n-x_0}=0 }[/math] ולכן [math]\displaystyle{ f'(x_0)=0 }[/math].
לכן [math]\displaystyle{ g'(f(x_0))\cdot f'(x_0)=0 }[/math].
כמו כן, [math]\displaystyle{ \frac{g(f(a_n))-g(f(x_0))}{a_n-x_0}=0 }[/math].
לכן בכל מקרה קיבלנו כי [math]\displaystyle{ \frac{g(f(x_n))-g(f(x_0))}{x_n-x_0}\to g'(f(x_0))\cdot f'(x_0) }[/math]
סה"כ [math]\displaystyle{ (g\circ f)'(x_0)=g'(f(x_0))\cdot f'(x_0) }[/math].

נגזרת של חזקה

עבור [math]\displaystyle{ x\gt 0 }[/math] מתקיים [math]\displaystyle{ (x^\alpha)'=(e^{ln\left(x^\alpha\right)})' = (e^{\alpha\cdot ln(x)})' = e^{\alpha\cdot ln(x)}\cdot \frac{\alpha}{x} = x^\alpha \cdot \frac{\alpha}{x} = \alpha x^{\alpha-1} }[/math]
דוגמא: חישוב הנגזרת של [math]\displaystyle{ x^x }[/math]

נגזרת מנה

תהיינה f,g גזירות בנקודה x כך ש [math]\displaystyle{ g(x)\neq 0 }[/math]:

נזכור כי [math]\displaystyle{ (\frac{1}{x})'=-\frac{1}{x^2} }[/math]
אזי בנקודה x מתקיים: [math]\displaystyle{ \left(\frac{f}{g}\right)'=\left(f\cdot \frac{1}{g}\right)' = f'\cdot \frac{1}{g} + f\cdot \frac{-g'}{g^2} = \frac{f'g-g'f}{g^2} }[/math]

פונקציות הופכיות ונגזרתן

פונקציות הפיכות (הוכחות והגדרות מדוייקות בבדידה).
- פונקציה [math]\displaystyle{ f:[a,b]\to [c,d] }[/math] הפיכה אם"ם היא חח"ע ועל
- הפונקציה ההופכית היא [math]\displaystyle{ f^{-1}:[c,d]\to[a,b] }[/math] ומתקיים כי [math]\displaystyle{ f(x)=y }[/math] אם"ם [math]\displaystyle{ x=f^{-1}(y) }[/math]

טענה: אם [math]\displaystyle{ f:[a,b]\to [c,d] }[/math] רציפה בקטע [math]\displaystyle{ [a,b] }[/math], אזי [math]\displaystyle{ f^{-1}:[c,d]\to[a,b] }[/math] רציפה בקטע [math]\displaystyle{ [c,d] }[/math].
- הוכחה:
- תהי [math]\displaystyle{ y_0\neq y_n\to y_0 }[/math], צ"ל ש [math]\displaystyle{ f^{-1}(y_n)\to f^{-1}(y_0) }[/math]
- יהי גבול חלקי [math]\displaystyle{ x_n=f^{-1}(y_n)\to L }[/math].
- אזי [math]\displaystyle{ f(x_n)=y_n\to y_0 }[/math].
- מצד שני, לפי רציפות הפונקציה f מתקיים [math]\displaystyle{ f(x_n)\to f(L) }[/math].
- לכן [math]\displaystyle{ f(L)=y_0 }[/math] ולכן [math]\displaystyle{ L=f^{-1}(y_0) }[/math].

טענה: תהי [math]\displaystyle{ f:[a,b]\to [c,d] }[/math] הפיכה ורציפה. ונניח כי היא גזירה בנק' [math]\displaystyle{ a\lt x_0\lt b }[/math] כך ש [math]\displaystyle{ f'(x_0)\neq 0 }[/math].

אזי [math]\displaystyle{ f^{-1} }[/math] גזירה בנק' [math]\displaystyle{ f(x_0) }[/math] ומתקיים כי

[math]\displaystyle{ (f^{-1})'(f(x_0))=\frac{1}{f'(x_0)} }[/math] או בנוסח אחר-

[math]\displaystyle{ (f^{-1})'(x)=\frac{1}{f'(f^{-1}(x))} }[/math]

- הוכחה:
- [math]\displaystyle{ (f^{-1})'(f(x_0)) = \lim_{y\to f(x_0)}\frac{f^{-1}(y)-f^{-1}(f(x_0))}{y-f(x_0)} }[/math]
- תהי [math]\displaystyle{ f(x_0)\neq y_n\to f(x_0) }[/math] ונסמן [math]\displaystyle{ x_n=f^{-1}(y_n) }[/math].
- אזי מתוך רציפות וחח"ע נובע כי [math]\displaystyle{ x_0\neq x_n\to f^{-1}(f(x_0))=x_0 }[/math]
- [math]\displaystyle{ \frac{f^{-1}(y_n)-f^{-1}(f(x_0))}{y_n-f(x_0)} = \frac{x_n-x_0}{f(x_n)-f(x_0)} \to \frac{1}{f'(x_0)} }[/math]

דוגמא חשובה:
[math]\displaystyle{ tan:(-\frac{\pi}{2},\frac{\pi}{2})\to\mathbb{R} }[/math] הפיכה וההופכית שלה נקראית [math]\displaystyle{ arctan }[/math].
[math]\displaystyle{ tan^2(x)+1 = \frac{sin^2(x)}{cos^2(x)}+1 = \frac{1}{cos^2(x)} }[/math]
[math]\displaystyle{ arctan'(x) = \frac{1}{\frac{1}{cos^2(arctan(x))}} = \frac{1}{tan^2(arctan(x))+1}=\frac{1}{1+x^2} }[/math]

פרק 6 - חקירה

משפטי חקירת פונקציות

משפט ערך הביניים.
תהי f רציפה ב[math]\displaystyle{ [0,1] }[/math] כך ש[math]\displaystyle{ f(1)=2 }[/math], הוכיחו שקיימת נק' [math]\displaystyle{ c\in [0,1] }[/math] עבורה [math]\displaystyle{ f(c)=\frac{1}{c} }[/math]
- נעביר אגף ונביט בפונקציה [math]\displaystyle{ h(x)=f(x)-\frac{1}{x} }[/math] שצריך למצוא שורש שלה.
- [math]\displaystyle{ h(1)\gt 0 }[/math].
- [math]\displaystyle{ \lim_{x\to 0^+}h(x)=f(0)-\infty=-\infty }[/math] ולכן קיימת נקודה [math]\displaystyle{ 0\lt d\lt 1 }[/math] עבורה [math]\displaystyle{ h(d)\lt 0 }[/math].
- לפי משפט ערך הביניים בקטע [math]\displaystyle{ [d,1] }[/math] קיימת נק' המאפסת את הפונקציה h.

משפטי ויירשטראס.
- פונקציה רציפה בקטע סופי סגור - חסומה.
- פונקציה רציפה בקטע סופי סגור - מקבלת מינימום ומקסימום.

משפט פרמה.
- אם פונקציה גזירה בנק' קיצון מקומי, הנגזרת שווה שם לאפס.
- ההפך אינו נכון.
משפט רול.
- פונקציה רציפה בקטע סגור, וגזירה בקטע הפתוח, שמקבלת את אותו ערך בקצוות - הנגזרת שלה מתאפסת בקטע הפתוח.
- לפולינום יש לכל היותר n שורשים שונים.
משפט לגראנז'.
- פונקציה רציפה בקטע סגור, וגזירה בקטע הפתוח מקבלת את השיפוע בין שתי נקודות הקצה בנגזרת בנק' כלשהי.
משפט לגראנז' המוכלל.
- שתי פונקציות רציפות בקטע סגור, גזירות בקטע הפתוח, והנגזרת של האחת אינה מתאפסת. אזי מנת הנגזרות שווה למנת השיפועים בנק' מסויימת.

הוכחת משפט לגראנז' המוכלל, שמוכיח גם את משפט לגראנז' עצמו כמקרה פרטי.
- ראשית, כיוון ש[math]\displaystyle{ g'(x)\neq 0 }[/math] בקטע [math]\displaystyle{ (a,b) }[/math] נובע לפי רול כי [math]\displaystyle{ g(a)\neq g(b) }[/math] ולכן מותר לחלק בהפרש ביניהם.
- [math]\displaystyle{ h(x)=f(x)-f(a) - \frac{f(b)-f(a)}{g(b)-g(a)}(g(x)-g(a)) }[/math]
- [math]\displaystyle{ h(a)=h(b)=0 }[/math] ולכן לפי רול קיימת נק' [math]\displaystyle{ c\in (a,b) }[/math] עבורה [math]\displaystyle{ h'(c)=0 }[/math] וזה מה שרצינו להוכיח.
- (שימו לב שמותר לחלק ב[math]\displaystyle{ g'(c) }[/math].)
- עבור [math]\displaystyle{ g(x)=x }[/math] נקבל את משפט לאגראנז' הרגיל.

קשר בין הנגזרת לפונקציה

פונקציה גזירה עולה אם"ם הנגזרת שלה גדולה או שווה אפס.
פונקציה עולה ממש אם"ם הנגזרת שלה גדולה או שווה אפס, ולא מתאפסת על קטע.

כלל לופיטל

כלל לופיטל (הוכחה לאפס חלקי אפס בנקודה סופית).
כיצד להעזר בלופיטל בכל אחד מהמקרים הבעייתיים.